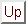


Next: 2.3 Galerkin's Method
Up: 2. Finite Element Method
Previous: 2.1 The Systems of
The Rayleigh-Ritz method seeks a stationary point of a variational functional.
For operators
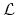 which are self-adjoint and positive-definite, the stationary point of the functional,
which are self-adjoint and positive-definite, the stationary point of the functional,
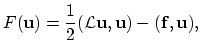 |
(2.4) |
is an exact solution of (2.1), assuming
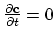 . In (2.4) the inner product of the two vector functions is defined as,
. In (2.4) the inner product of the two vector functions is defined as,
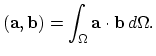 |
(2.5) |
The functional
 reaches a minimum for the function
reaches a minimum for the function
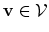 , if the first variation
, if the first variation 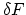 is zero for this function, or equivalently,
is zero for this function, or equivalently,
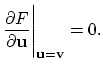 |
(2.6) |



Next: 2.3 Galerkin's Method
Up: 2. Finite Element Method
Previous: 2.1 The Systems of
H. Ceric: Numerical Techniques in Modern TCAD
![]() which are self-adjoint and positive-definite, the stationary point of the functional,
which are self-adjoint and positive-definite, the stationary point of the functional,