2.2.2.2 Diffusion Current

The component of the current which is caused by the thermal motion of the carriers is called diffusion current. It is driven by a gradient in the carrier concentration. The law of diffusion which originally stems from the theory of dilute gases reads

$\displaystyle \ensuremath{\boldsymbol{\mathrm{F}}}_n$	$\displaystyle = - D_n \, \ensuremath{\ensuremath{\ensuremath{\boldsymbol{\mathrm{\nabla}}}}\, n}\ ,$	(2.29)
$\displaystyle \ensuremath{\boldsymbol{\mathrm{F}}}_p$	$\displaystyle = - D_p \, \ensuremath{\ensuremath{\ensuremath{\boldsymbol{\mathrm{\nabla}}}}\, p}\ ,$	(2.30)

where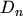

and

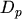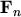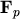are the diffusion coefficients for electrons and holes, respectively, $\ensuremath{\boldsymbol{\mathrm{F}}}_n$ and $\ensuremath{\boldsymbol{\mathrm{F}}}_p$ are the respective particle flux densities, which have to be multiplied by the charge of the particle to get the electrical current density

$\displaystyle \ensuremath{\boldsymbol{\mathrm{J}}}^\mathrm{diffusion}_n$	$\displaystyle = -$	$\displaystyle \mathrm{q}\,$	$\displaystyle \ensuremath{\boldsymbol{\mathrm{F}}}_n \ ,$	(2.31)
$\displaystyle \ensuremath{\boldsymbol{\mathrm{J}}}^\mathrm{diffusion}_p$	$\displaystyle =$	$\displaystyle \mathrm{q}\,$	$\displaystyle \ensuremath{\boldsymbol{\mathrm{F}}}_p \ .$	(2.32)

For conditions close to thermal equilibrium and for non-degenerate carrier systems (BOLTZMANN statistics), the diffusion coefficients are related to the mobilities by the EINSTEIN relation

$\displaystyle D_n = \mu_n \, \frac{\mathrm{k}_\mathrm{B}\, T_n}{\mathrm{q}} \ ,$	(2.33)
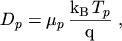$\displaystyle D_p = \mu_p \, \frac{\mathrm{k}_\mathrm{B}\, T_p}{\mathrm{q}} \ ,$	(2.34)

where 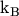$\mathrm{k}_\mathrm{B}$ is BOLTZMANN's constant.

Superposition of the current components yields the drift-diffusion current relations

$\displaystyle \ensuremath{\boldsymbol{\mathrm{J_n}}}$	$\displaystyle = \mathrm{q}\, n \, \mu_n \, \ensuremath{\boldsymbol{\mathrm{E}}}$		$\displaystyle + \mathrm{q}\, D_n \, \ensuremath{\ensuremath{\ensuremath{\boldsymbol{\mathrm{\nabla}}}}\, n}\ ,$	(2.35)
$\displaystyle \ensuremath{\boldsymbol{\mathrm{J_p}}}$	$\displaystyle = \mathrm{q}\, p \, \mu_p \, \ensuremath{\boldsymbol{\mathrm{E}}}$		$\displaystyle - \mathrm{q}\, D_p \, \ensuremath{\ensuremath{\ensuremath{\boldsymbol{\mathrm{\nabla}}}}\, p}\ .$	(2.36)

M. Gritsch: Numerical Modeling of Silicon-on-Insulator MOSFETs PDF


Previous: 2.2.2.1 Drift Current Up: 2.2.2 Drift-Diffusion Transport Model Next: 2.2.3 Carrier Continuity Equations