In the current equation (2.76) a temperature tensor occurs. The
diagonal components of this tensor are defined as
and the temperature 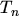 used in the energy transport model is defined through the mean carrier energy
(cf. eqn. (2.94))
used in the energy transport model is defined through the mean carrier energy
(cf. eqn. (2.94))
 |
(5.4) |
For parabolic bands the relation
 |
(5.5) |
holds.
Monte Carlo simulations of a one-dimensional  -
-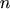 -
- test structure show that the
temperature components parallel (
test structure show that the
temperature components parallel (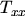 ) and normal (
) and normal (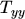 ) to the direction of the
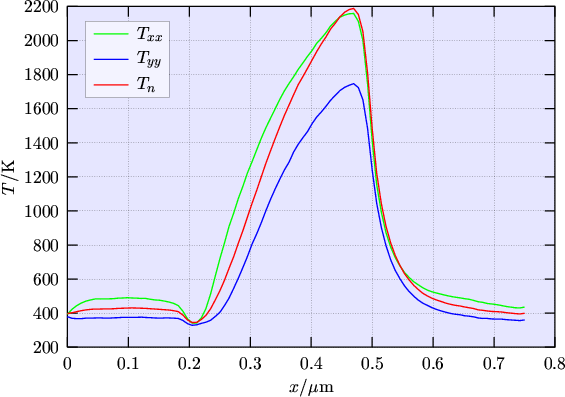
current flow are quite different (Fig. 5.4). In particular, the transverse
temperature component is smaller than the longitudinal component [64, Fig.7].
This encourages the derivation of a transport model which takes an anisotropic distribution function into account.
) to the direction of the
current flow are quite different (Fig. 5.4). In particular, the transverse
temperature component is smaller than the longitudinal component [64, Fig.7].
This encourages the derivation of a transport model which takes an anisotropic distribution function into account.
Figure 5.4:
Components of the temperature tensor compared to the temperature 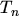 from the
mean energy obtained by Monte Carlo simulations.
from the
mean energy obtained by Monte Carlo simulations.
|
 |
M. Gritsch: Numerical Modeling of Silicon-on-Insulator MOSFETs PDF
![]() -
-![]() -
-![]() test structure show that the
temperature components parallel (
test structure show that the
temperature components parallel (![]() ) and normal (
) and normal (![]() ) to the direction of the
current flow are quite different (Fig. 5.4). In particular, the transverse
temperature component is smaller than the longitudinal component [64, Fig.7].
This encourages the derivation of a transport model which takes an anisotropic distribution function into account.
) to the direction of the
current flow are quite different (Fig. 5.4). In particular, the transverse
temperature component is smaller than the longitudinal component [64, Fig.7].
This encourages the derivation of a transport model which takes an anisotropic distribution function into account.
