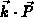 model and the Wentzel-Kramers-Brillouin-Jeffreys (WKBJ)
approximation.
model and the Wentzel-Kramers-Brillouin-Jeffreys (WKBJ)
approximation.




Direct Tunneling Rate in Spatially Variable Fields
In this appendix, an approximate expression for the number of the electron-hole
pairs generated by internal field emission in a linearly variable electric field
is derived, assuming direct tunneling. The expression is based on the two-band
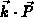 model and the Wentzel-Kramers-Brillouin-Jeffreys (WKBJ)
approximation.
model and the Wentzel-Kramers-Brillouin-Jeffreys (WKBJ)
approximation.
We consider direct tunneling process. Direct tunneling is important in direct
gap semiconductors like InSb, Hg Cd
Cd Te, GaAs, GaSb,
Ga
Te, GaAs, GaSb,
Ga Al
Al As, InGaAs, InAlGaAs, Pb
As, InGaAs, InAlGaAs, Pb Se
Se Te
[518][55][54][7]. In Si direct tunneling
is negligible in comparison with indirect (phonon-assisted) tunneling for all
practically interesting fields (
Te
[518][55][54][7]. In Si direct tunneling
is negligible in comparison with indirect (phonon-assisted) tunneling for all
practically interesting fields (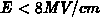
 ) and temperatures. This is a consequence of very large direct band
gap of Si
) and temperatures. This is a consequence of very large direct band
gap of Si 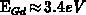 in comparison with the (indirect)
band gap
in comparison with the (indirect)
band gap 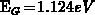 [86]. In Ge, both, direct and
indirect tunneling are important because of low direct band gap
[86]. In Ge, both, direct and
indirect tunneling are important because of low direct band gap
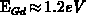 in comparison with the indirect band gap
in comparison with the indirect band gap
 ; indirect tunneling is important at low fields and
direct tunneling at high fields. Although direct tunneling is negligible in
Si-devices in practice, we think that the conclusions obtained in
Section 4.2 by using the direct tunneling model which is presented
in this appendix are qualitatively applicable to dominant phonon-assisted
tunneling.
; indirect tunneling is important at low fields and
direct tunneling at high fields. Although direct tunneling is negligible in
Si-devices in practice, we think that the conclusions obtained in
Section 4.2 by using the direct tunneling model which is presented
in this appendix are qualitatively applicable to dominant phonon-assisted
tunneling.
In order to model band-to-band tunneling in MOSFETs either some result from the
junction-tunneling theory [430][237][88][7] or from the
theory of internal field emission in an infinite
medium [525][430][244][236][212][13] has been applied in the
literature. In the junction-tunneling theory, the flux of the quasi-free moving
valence band electrons which are described by the Bloch wave functions incidents
on the potential barrier (junction). The main quantity of interest is the
transmission probability of one electron from the valence to the conduction
band, 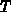 . Dependent on the approach employed, the transmission probability is
either a function of the energy for the motion in the tunneling direction
. Dependent on the approach employed, the transmission probability is
either a function of the energy for the motion in the tunneling direction
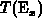 , of the energy associated with the motion perpendicular to the
tunneling direction
, of the energy associated with the motion perpendicular to the
tunneling direction 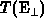 , or of the both energies
, or of the both energies
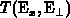 [109]. On the other hand, in the
internal field emission theory the electrons oscillate across the valence band
due to applied field. The basic quantity of interest is the probability per unit
time of the transition of an electron from the valence to the conduction band
[109]. On the other hand, in the
internal field emission theory the electrons oscillate across the valence band
due to applied field. The basic quantity of interest is the probability per unit
time of the transition of an electron from the valence to the conduction band
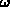 :
:
Expression H.1 originates from the Fermi Golden Rule. 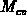 is the interband matrix element for the transition between the states of equal
energy in the valence and the conduction band,
is the interband matrix element for the transition between the states of equal
energy in the valence and the conduction band,  is the electric field
strength and
is the electric field
strength and 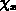 is the width of the intersection of the I Brillouin
zone in the field direction (here we assume that
is the width of the intersection of the I Brillouin
zone in the field direction (here we assume that 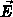 is parallel to
is parallel to
 -direction). The total number of generated pairs follows after an integration
over the normal impulse component
-direction). The total number of generated pairs follows after an integration
over the normal impulse component 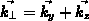
 is the number of the valence band electrons per unit cell interesting for
tunneling (
is the number of the valence band electrons per unit cell interesting for
tunneling (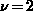 for simple cubic lattice, due to spin). Note that we
consider the planar problem in three dimensions.
for simple cubic lattice, due to spin). Note that we
consider the planar problem in three dimensions.
In our opinion, neither the expressions known from the junction tunneling
problem nor those in the internal field emission theory can be applied to the
band-to-band tunneling problem in MOSFETs because of the vicinity of the
oxide/bulk interface. There is a strong quantization of the motion in direction
perpendicular to the gate/oxide interface in the potential well induced by the
high transversal field. For tunneling in the gate/drain overlap region in
 -channel MOSFETs the initial states are strongly quantized, while the final
states are like those in the diode-tunneling problem. The opposed conditions
hold in
-channel MOSFETs the initial states are strongly quantized, while the final
states are like those in the diode-tunneling problem. The opposed conditions
hold in  -channel devices. This effect is not accounted for, neither in the
literature nor in the present derivation. We think, however, that a proper
model of tunneling in MOSFETs must include the interface-vicinity effect. In
the following, the internal field emission theory for an infinite medium is
applied.
-channel devices. This effect is not accounted for, neither in the
literature nor in the present derivation. We think, however, that a proper
model of tunneling in MOSFETs must include the interface-vicinity effect. In
the following, the internal field emission theory for an infinite medium is
applied.
Let us assume that the electric field changes according to
where 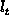 is the tunneling length (Figure H.1). The interband
matrix element
is the tunneling length (Figure H.1). The interband
matrix element 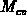 can be derived by employing the time-independent
perturbation theory, as done in [236] for uniform field. It reads
can be derived by employing the time-independent
perturbation theory, as done in [236] for uniform field. It reads
where
is the interband matrix element for the potential energy operator 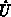 and
and
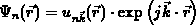 (
( stands for
stands for  or
or  ) are Bloch wave functions. For
field H.3, the potential energy operator in the impulse
representation becomes
) are Bloch wave functions. For
field H.3, the potential energy operator in the impulse
representation becomes
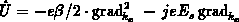 ,
where we substituted the
,
where we substituted the  -position operator
-position operator 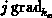 .
The
.
The 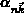 , with
, with  and
and  , are eigenfunctions in the
conduction and the valence band in the presence of the electric field. From the
time-independent Schrödinger equation
, are eigenfunctions in the
conduction and the valence band in the presence of the electric field. From the
time-independent Schrödinger equation
it follows
width
being the intraband correction of the band-edge energy. Of course,
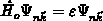 is fulfilled for the
unperturbed Hamiltonian
is fulfilled for the
unperturbed Hamiltonian 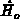 and the Bloch wave functions
and the Bloch wave functions
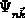 .
.
After adopting a band model, the dispersion relation 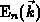 is
known, as well as
is
known, as well as 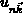 . Consequently,
. Consequently, 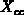 ,
, 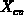 ,
, 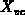 and
and
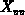 can be calculated. The eigenfunctions follow from H.7
by neglecting the interband term, i.e. the second term on the
left-hand-side, after applying the periodic boundary conditions at the edges
of the I Brillouin zone. Finally,
can be calculated. The eigenfunctions follow from H.7
by neglecting the interband term, i.e. the second term on the
left-hand-side, after applying the periodic boundary conditions at the edges
of the I Brillouin zone. Finally, 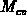 can be calculated by
using H.4.
can be calculated by
using H.4.
Following this standard approach ([236][109][13]), the authors
have derived in [457][456][56] the interband matrix element
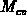 for linearly variable field H.3, assuming two-band
for linearly variable field H.3, assuming two-band
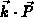 model. It has been obtained that the field variation (the
parameter
model. It has been obtained that the field variation (the
parameter 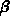 ) does not influence the exponent in the expression for the
number of the generated pairs
) does not influence the exponent in the expression for the
number of the generated pairs 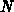 . The generation rate was only weakly
influenced by
. The generation rate was only weakly
influenced by 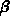 due to the preexponential dependence. This result
seems to be not justified from the physical point of view, because the field
variation directly changes the tunneling length. Since the wave functions decay
exponentially within the forbidden gap, the change in the tunneling length
should influence
due to the preexponential dependence. This result
seems to be not justified from the physical point of view, because the field
variation directly changes the tunneling length. Since the wave functions decay
exponentially within the forbidden gap, the change in the tunneling length
should influence 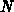 in an exponential manner. The erroneous result in these
papers we attribute to the assumption that the eigenfunctions
in an exponential manner. The erroneous result in these
papers we attribute to the assumption that the eigenfunctions
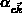 and
and  do not change explicitly with the
electric field strength along the tunneling path. A small field variation has
been implicitly adopted in the derivation. This assumption is not justified. In
addition, the derivations in [457][456][56] employ the result of
an analytical integration over the parallel impulse component
do not change explicitly with the
electric field strength along the tunneling path. A small field variation has
been implicitly adopted in the derivation. This assumption is not justified. In
addition, the derivations in [457][456][56] employ the result of
an analytical integration over the parallel impulse component 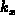 , which has
been proposed in [54] while solving the uniform field problem. We have
proven that this analytical result is incorrect. Moreover, we have obtained that
Kane approximation is an accurate solution to the problem [236].
, which has
been proposed in [54] while solving the uniform field problem. We have
proven that this analytical result is incorrect. Moreover, we have obtained that
Kane approximation is an accurate solution to the problem [236].
In order to overcome the difficulties in analytical derivation of the rigorous result, we apply the WKBJ method with an appropriate barrier for the variable field to derive an approximate model. We follow the quasi-classical interpretation of the internal field emission result by introducing a quantity ([13])
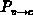 is the probability of the penetration of an incident electron from
the valence band to the conduction band and
is the probability of the penetration of an incident electron from
the valence band to the conduction band and  is the period of
oscillations of the valence band electrons. Relationship H.9
assumes a homogenous field. The total number of pairs generated by internal
field emission results by replacing H.9 in H.2
is the period of
oscillations of the valence band electrons. Relationship H.9
assumes a homogenous field. The total number of pairs generated by internal
field emission results by replacing H.9 in H.2
Expression H.10 may be written in the form
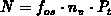 , where
, where 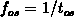 is the field-dependent
oscillation frequency,
is the field-dependent
oscillation frequency, 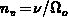 is the concentration of the
valence band electrons which are active in tunneling and
is the concentration of the
valence band electrons which are active in tunneling and 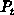 is the total
averaged penetration probability per particle ([525][302]). Let us
introduce the transmission probability per particle calculated by WKBJ
approximation,
is the total
averaged penetration probability per particle ([525][302]). Let us
introduce the transmission probability per particle calculated by WKBJ
approximation, 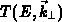 . As well known, the transmission
probability reads
. As well known, the transmission
probability reads
where 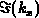 is the imaginary part of the wave vector in direction of the
electric field. The
is the imaginary part of the wave vector in direction of the
electric field. The  and
and 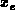 denote the classical turning points. When
denote the classical turning points. When
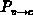 is calculated from
is calculated from 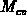 by using H.9 where
by using H.9 where
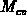 follows from the time-independent perturbation theory assuming the
follows from the time-independent perturbation theory assuming the
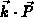 band model and a constant field
band model and a constant field  , the following
relationship can be established [237]
, the following
relationship can be established [237]
Consequently, in a uniform field the WKBJ yields the same result as the
rigorous approach, with exception of the preexponential factor
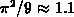 . Motivated by this fact and the discussion
in [237] we heuristically assume
. Motivated by this fact and the discussion
in [237] we heuristically assume
for a spatially variable field.
For the band model, a two-band 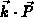 model is adopted, for
which it is known to result in a dispersion relation
model is adopted, for
which it is known to result in a dispersion relation
for the conduction band and
for the valence band, where 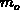 is the free electron mass in vacuum,
is the free electron mass in vacuum,
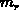 is the reduced mass in tunneling
is the reduced mass in tunneling 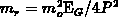 and
and 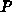 is
the matrix element for the interaction between both bands. We further consider
the kinetic energy in the conduction band H.14 (
is
the matrix element for the interaction between both bands. We further consider
the kinetic energy in the conduction band H.14 (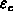 is replaced by
is replaced by  ), which yields for the factor
), which yields for the factor 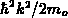
The physical solution with ``+'' sign is chosen so that in the limit
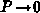 ,
, 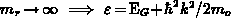 is fulfilled
is fulfilled . For a typical condition
. For a typical condition
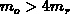 a very accurate approximation of H.16 may follow
a very accurate approximation of H.16 may follow
In the conditions  relationship H.17 reduces to
relationship H.17 reduces to
which also follows by neglecting the ``free-kinetic energy'' term
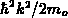 in H.14. Expression H.18,
known as Franz's dispersion relation [91], is sometimes a crude
approximation of H.16, but is very convenient for analytical
handling and will be assumed henceforward. The
in H.14. Expression H.18,
known as Franz's dispersion relation [91], is sometimes a crude
approximation of H.16, but is very convenient for analytical
handling and will be assumed henceforward. The 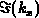 required
for H.11 follows from H.18, with benefit of
required
for H.11 follows from H.18, with benefit of

where 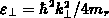 is the energy associated
with the motion in direction perpendicular to the field.
is the energy associated
with the motion in direction perpendicular to the field.
A particle with an incident energy 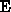 changes its impulse
changes its impulse 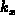 while
penetrating into the forbidden gap according to the variation in the relative
potential energy
while
penetrating into the forbidden gap according to the variation in the relative
potential energy 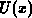 due to the field
due to the field 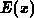
At the incident point 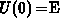 and
and 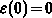 ,
while
,
while 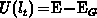 and
and 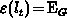 hold at the endpoint, Figure H.1. The equivalent tunneling
barrier
hold at the endpoint, Figure H.1. The equivalent tunneling
barrier 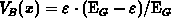 has an
interesting property
has an
interesting property
Therefore, the particles properly feel the forces 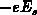 and
and 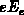 at the
band edges.
at the
band edges.
Field H.3 yields
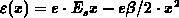 . The barrier
. The barrier 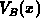 has a
maximum of
has a
maximum of 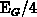 at
at
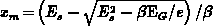 . Henceforward
we replace
. Henceforward
we replace  with
with 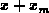 . It follows
. It follows
The barrier 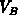 is shown in Figure H.2 for different
field variation
is shown in Figure H.2 for different
field variation 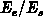 as a parameter.
The classical turning points in the WKBJ approach, including the normal-impulse
energy
as a parameter.
The classical turning points in the WKBJ approach, including the normal-impulse
energy 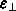 , are given by
, are given by
where  . Further
useful relationships are
. Further
useful relationships are 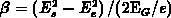 ,
,
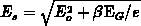 and
and
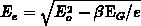 .
.
After a replacement of H.22 in H.19, transmission probability H.11 reads
where
The subintegral function in 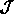 posses a restriction
posses a restriction
which also follows from the criterium that 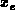 in H.23 is a
finite real number.
The integral
in H.23 is a
finite real number.
The integral 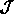 can be transformed
can be transformed
with
Finally,
In a constant field  it follows
it follows 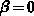 ,
, 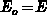 ,
, 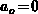 and
and 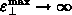 . Relationship H.29
yields
. Relationship H.29
yields
This expression is identical to the Kane formula in [236], with
exception for the prefactor 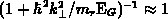 .
Relationship H.29 is replaced in H.10. We
integrate over the energy
.
Relationship H.29 is replaced in H.10. We
integrate over the energy 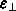 instead of
instead of 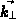 ,
with benefit of
,
with benefit of 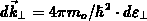 .
The integration interval ranges from
.
The integration interval ranges from 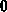 to
to 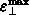 given
by H.26. In order to simplify the expression let us observe that
given
by H.26. In order to simplify the expression let us observe that
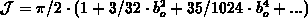 is a
rapidly convergent series expansion, because of restriction H.26
which means
is a
rapidly convergent series expansion, because of restriction H.26
which means 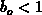 . We truncate this expansion after the second term with
. We truncate this expansion after the second term with
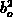 and keep the first three terms in the exponent of H.29
til the first power of
and keep the first three terms in the exponent of H.29
til the first power of 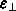 . In order to
integrate H.10, it is assumed that
. In order to
integrate H.10, it is assumed that
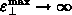 . The resulting
. The resulting 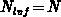 reads
reads
It is assumed that the oscillation frequency  depends on the field at
the starting point
depends on the field at
the starting point 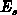 . In a uniform field
. In a uniform field 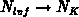 , where
, where 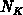 is
the Kane-Keldysh result (expression (39) in [236]).
is
the Kane-Keldysh result (expression (39) in [236]).
The replacement 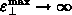 is justified if the
exponential term for
is justified if the
exponential term for 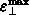 is larger than about
is larger than about 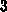 . It
follows
. It
follows
where we benefit from H.26. It is adopted that the characteristic
field 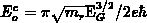 is much larger than the
field of interest
is much larger than the
field of interest 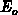 . For e.g.
. For e.g. 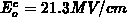 and
and 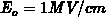 it
follows
it
follows 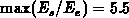 , whereas for
, whereas for 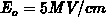 we have
we have
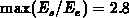 . Consequently, for common electric field strengths
the proposed expression holds for the field variations of practical interest.
. Consequently, for common electric field strengths
the proposed expression holds for the field variations of practical interest.
In Figure H.3 we compared the expression for 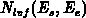 with
with 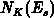 and
and 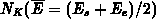 assuming a simple
one-dimensional analytical model for the potential and field distributions in
the totally depleted uniformly doped bulk. The tunneling rate from the surface
is plotted against the surface field
assuming a simple
one-dimensional analytical model for the potential and field distributions in
the totally depleted uniformly doped bulk. The tunneling rate from the surface
is plotted against the surface field 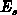 which is also the starting field in
tunneling.
which is also the starting field in
tunneling.



