3.6.1 Strain-Induced Conduction Band Splitting
In this section we give expressions for the strain-induced energy shifts of the
nondegenerate energy levels of the conduction band edges of the cubic crystal class
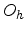 .
.
At the  and
and 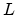 point, and along the
point, and along the 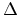 symmetry line, the
deformation potential operators
symmetry line, the
deformation potential operators
 are scalars and
given by one ore two independent constants. Neglecting the strain-induced
splitting of the degenerate conduction bands
are scalars and
given by one ore two independent constants. Neglecting the strain-induced
splitting of the degenerate conduction bands 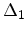 and
and
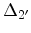 at the
at the  point
for the moment, the energy shifts of the conduction band edge of valleys along
the
point
for the moment, the energy shifts of the conduction band edge of valleys along
the
 and
and
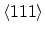 direction can be calculated
from two independent deformation potential constants [Balslev66]
direction can be calculated
from two independent deformation potential constants [Balslev66]
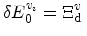 Tr Tr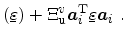 |
(3.47) |
Here,
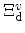 denotes the dilatation- and
denotes the dilatation- and
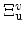 the uniaxial
deformation potential constant for a valley of type
the uniaxial
deformation potential constant for a valley of type
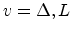 , and
, and
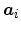 is a unit vector parallel to the
is a unit vector parallel to the
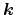 vector of valley
vector of valley
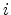 . The valley shift of the
. The valley shift of the  conduction band minimum can be
obtained from a single deformation potential constant
conduction band minimum can be
obtained from a single deformation potential constant
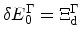 Tr Tr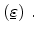 |
(3.48) |
The valley splitting from uniaxial stress along any direction can be obtained
from the strain tensor using the relations above. The strain tensors resulting
from uniaxial stress are discussed in Section 3.3.2. The
analytical expressions for the energy shifts of the conduction band valleys for
three stress directions [100], [110], and [111] are given in in
Table 3.4.
Table 3.4:
Strain-induced energy shifts of the conduction
band valleys of cubic semiconductors when uniaxial stress 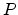 is applied along three high symmetry directions. The energy shifts are divided by
is applied along three high symmetry directions. The energy shifts are divided by 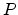 .
.
| stress direction |
valley |
valley direction |
 |
![$ [100]$](img292.png) |
 |
![$ [100]$](img292.png) |
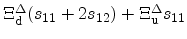 |
| |
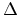 |
![$ [010][001]$](img410.png) |
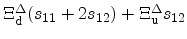 |
| |
 |
![$ [111][11\bar{1}][1\bar{1}1][\bar{1}11]$](img412.png) |
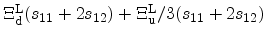 |
| |
 |
![$ [000]$](img414.png) |
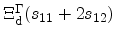 |
![$ [110]$](img416.png) |
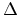 |
![$ [100][010]$](img417.png) |
 |
| |
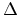 |
![$ [001]$](img296.png) |
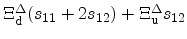 |
| |
 |
![$ [111][11\bar{1}]$](img419.png) |
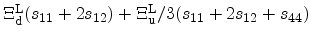 |
| |
 |
![$ [\bar{1}11][1\bar{1}1]$](img421.png) |
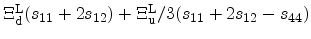 |
| |
 |
![$ [000]$](img414.png) |
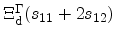 |
![$ [111]$](img14.png) |
 |
![$ [100][010][001]$](img423.png) |
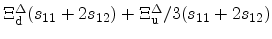 |
| |
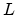 |
![$ [111]$](img14.png) |
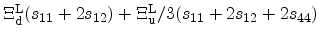 |
| |
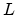 |
![$ [\bar{1}11][1\bar{1}1][11\bar{1}]$](img426.png) |
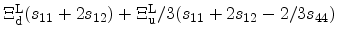 |
| |
 |
![$ [000]$](img414.png) |
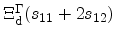 |
| |
|
|
|
|
E. Ungersboeck: Advanced Modelling Aspects of Modern Strained CMOS Technology
![]() and
and ![]() point, and along the
point, and along the ![]() symmetry line, the
deformation potential operators
symmetry line, the
deformation potential operators
![]() are scalars and
given by one ore two independent constants. Neglecting the strain-induced
splitting of the degenerate conduction bands
are scalars and
given by one ore two independent constants. Neglecting the strain-induced
splitting of the degenerate conduction bands ![]() and
and
![]() at the
at the ![]() point
for the moment, the energy shifts of the conduction band edge of valleys along
the
point
for the moment, the energy shifts of the conduction band edge of valleys along
the
![]() and
and
![]() direction can be calculated
from two independent deformation potential constants [Balslev66]
direction can be calculated
from two independent deformation potential constants [Balslev66]