The Gauß's law for magnetism (4.4) is satisfied by
introduction of the vector potential
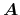 as:
as:
Inserting (4.5) into (4.2) yields
Substituting this into the relation of the electric displacement and the electric field
 |
(4.7) |
results into
The permittivity is considered to be homogenous, therefore the first
term in (4.8) is zero due to the definition of
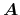 (4.5), so the conventional form of the
Poisson equation is obtained:
(4.5), so the conventional form of the
Poisson equation is obtained:
The space charge density
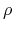 can be expressed as the product of the
elementary charge
can be expressed as the product of the
elementary charge
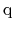 and the sum of the electron
and the sum of the electron 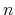 and hole
and hole 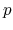 concentrations and the net concentration of ionized dopants
concentrations and the net concentration of ionized dopants
 :
:
Substituting (4.10) into (4.9) gives:
S. Vitanov: Simulation of High Electron Mobility Transistors