 |
|
||||
BiographyHans Kosina received the Diplomingenieur degree in electrical engineering and PhD from the Technische Universität Wien in 1987 and 1992, respectively. He was with the Institute of Flexible Automation at the Technische Universität Wien for one year and then joined the Institute for Microelectronics, where he is currently an associate professor. He received the venia docendi in microelectronics in 1998. In the summer of 1993, he was a visiting scientist at Motorola Inc., Austin, Texas, and in the summer of 1999, a visiting scientist at Intel Corp., Santa Clara, California. Dr. Kosina was chairman of the ''11th International Workshop on Computational Electronics'' held in Vienna in May 2006 and is a member of the advisory board of the International Workshop on Computational Nanotechnology. He has served as an Associate Editor of the IEEE Transactions on Computer-Aided Design of Circuits and Systems and the Journal of Computational Electronics. His current research interests include nanoelectronic device modeling and the development of numerical methods and algorithms for classical and quantum transport calculations. |
|||||
Carrier Transport in Nanostructured Semiconductor Devices
The three main topics under investigation include charge transport modeling in the presence of electron-electron scattering, numerical methods for the stationary Wigner and the von Neumann equation, and quantum transport models for device TCAD. The Boltzmann equation becomes nonlinear if electron-electron scattering (EES) is included in the scattering operator. On the other hand, a two-particle kinetic equation will remain linear in this case. A two-particle Monte Carlo method developed previously is based on calculating trajectory pairs and sampling the six-dimensional momentum-space of two particles. This year, we addressed the problem of statistical enhancement. The related algorithms aim to alter the distribution of sampling points to favor high-energy samples without affecting mean values. For single-particle simulations, the concept of particle splitting exists. However, we have discovered that this method is not directly applicable to the two-particle algorithm. Thus, we developed a new algorithm based on repeating those time frames that contain rare simulation events. Simulation experiments clearly show that the mean values remain unchanged, whereas the distribution of sampling points shifts to higher energies. The model equations for EES are generally derived for parabolic bands. Only in this special case can the transition rate be integrated analytically. We have extended the formalism to account for non-parabolic bands. The system of equations for energy and momentum conservation can still be solved analytically, whereas some approximations have to be made for the scattering rate. It turned out that satisfying the conservation laws in a simulation is crucial, whereas the exact value of the EES scattering is not critical. Figure 1 and Figure 2 show the energy distribution of electrons at the surface of a MOSFET. The non-parabolicity correction makes the electron energy relax faster in the drain region. A project on numerical methods for the stationary Wigner and von Neumann equations has continued. Bifurcation points in IV curves were discovered in simulation results using the self-consistent solver for the sigma equation and the von Neumann equation in a rotated coordinate system. The existence of bifurcation points was confirmed by solving the Schrödinger-Poisson system. Furthermore, we investigated numerical solution methods for the sigma equation in two dimensions. A 2D sigma solver with inflow boundary conditions has been implemented. In order to demonstrate consistency for non-trivial examples that require a larger mesh size, the solver has been parallelized using domain decomposition. In the simplest variant, an inflow problem is solved on each subdomain. The outflow from one subdomain is matched with the inflow from a neighboring subdomain. The over-determination problem appears in the sigma-equation in the conditions at the s-boundaries and in the Wigner equation by the conditions that must be imposed at k=0. These conditions can only be satisfied approximately but with any desired degree of accuracy. The concept of absorbing layers, which has proven successful in the case of the sigma-equation, has been transferred to the Wigner equation. The imaginary damping potential that is added in the s-domain transforms into a symmetric operator in the k-domain. Polynomial potentials of even degree were shown to work quite well. They have the desired property of a steep monotonous increase at large s, which effectively dampens the solution. In contrast to Frensley's upwind discretization, which does not show numerical convergence upon grid refinement, we can use symmetric finite differences. Our method allows us to use k-meshes with both an odd number of points (k=0 is included) and an even number (k=0 excluded as required by Frensly's method).
This year, a project dealing with quantum transport models for device TCAD has ended. An NEGF transport model in mode space representation, including electron-phonon scattering, has been implemented and coupled with the GTS Nano Device Simulator (NDS). If only one phonon energy is present, the energy grid decouples into several sub-grids. This feature has been exploited for parallelization and to reduce memory requirements. Carbon nanotube field-effect transistors (CNT FETs) have been chosen as benchmark devices for comparative simulations using the new NEGF solver and the existing subband-Boltzmann solver.
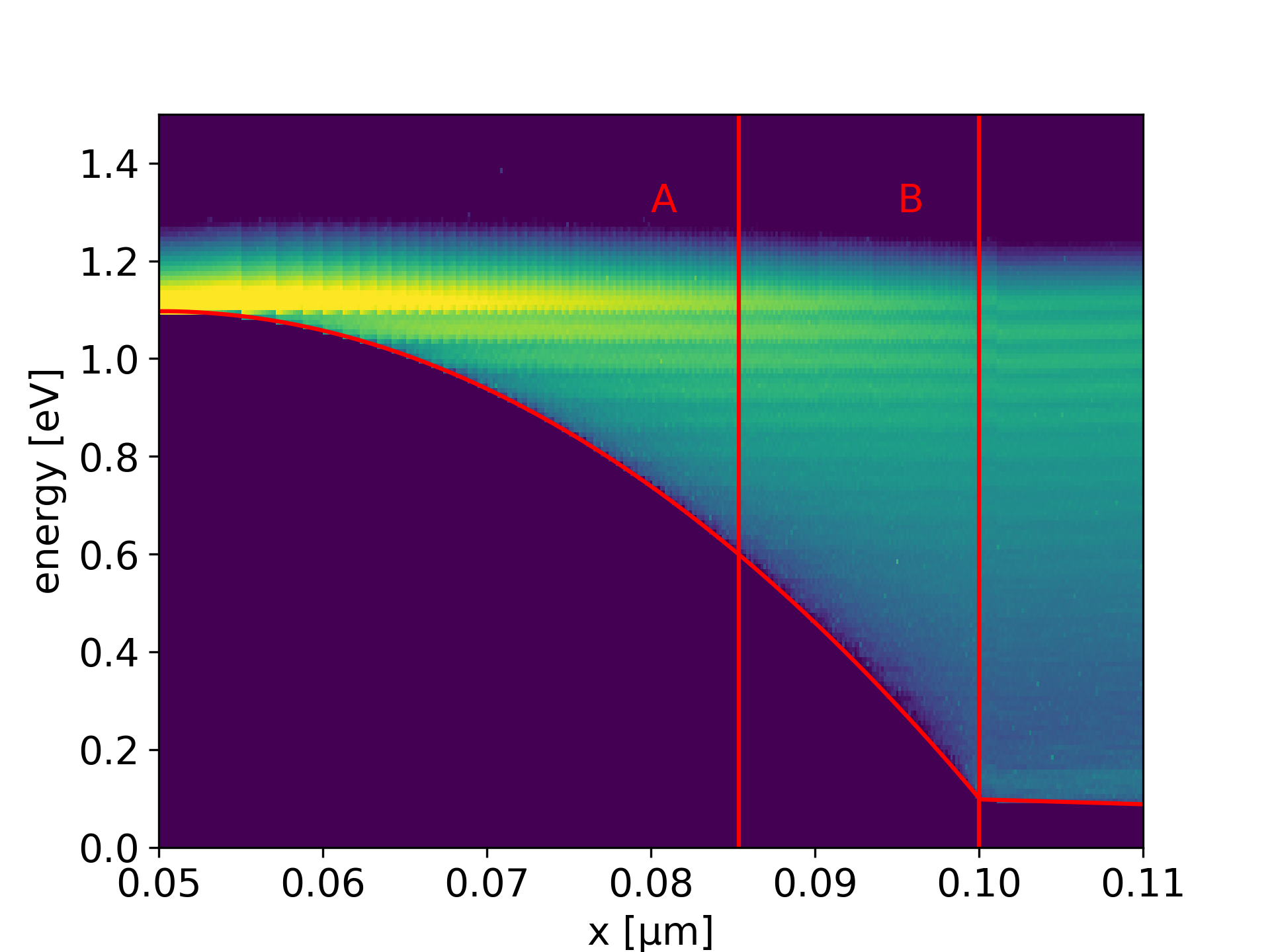
Fig. 1: Electron energy distribution function on the surface of a MOSFET for parabolic bands.

Fig. 2: Electron energy distribution function on the surface of a MOSFET for parabolic bands.


