The electrical permittivity tensor
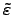 describes how the
electric field
describes how the
electric field
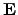 is related to the electric flux density
is related to the electric flux density
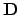 ,
,
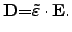 |
(3.10) |
For isotropic materials, the permittivity tensor reduces to a scalar-valued
quantity and the direction of the electric flux density and the electric field
is the same.
Hence, for isotropic materials the electrical permittivity can be approximated
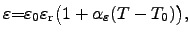 |
(3.11) |
where the temperature coefficient
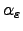 is determined by
is determined by
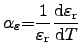 |
(3.12) |
and can be derived from the
CLAUSIUS3.1-MOSOTTI3.2equation [140]
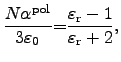 |
(3.13) |
where 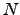 is the dipole density,
is the dipole density,
 the polarizability, and
the polarizability, and
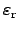 the relative permittivity.
This equation describes the relative permittivity as an implicit function of
the polarizability and the dipole density. The latter is an inherent property of
the material and does normally not change as long as the phase stage is
not altered.
Hence, the derivative with respect to the temperature
the relative permittivity.
This equation describes the relative permittivity as an implicit function of
the polarizability and the dipole density. The latter is an inherent property of
the material and does normally not change as long as the phase stage is
not altered.
Hence, the derivative with respect to the temperature 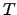 is
is
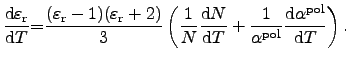 |
(3.14) |
Due to mass conservation, the thermal volume expansion can be assumed to be
equal to the negative temperature coefficient of the dipole density because the
number of atom inside a atomic unit cell remains constant.
The only assumption here is that the material persists in the phase stage.
Hence,
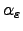 is
is
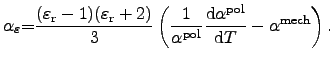 |
(3.15) |
According to the magnitude of the relative dielectric constant, materials can be
divided into two groups:
high- materials, which have a larger
materials, which have a larger
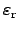 than
than
 , and
low-
, and
low- materials, which have a lower
materials, which have a lower
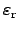 number.
Materials with higher relative permittivities than
number.
Materials with higher relative permittivities than
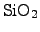 have a higher
dielectric displacement field (electric flux density) as
have a higher
dielectric displacement field (electric flux density) as
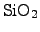 if the same
electric field is applied. Hence, the capacitive
coupling between the two opposite sides of the materials is much tighter as with
if the same
electric field is applied. Hence, the capacitive
coupling between the two opposite sides of the materials is much tighter as with
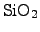 . Therefore, such materials are used to increase the capacitance
where the thickness of the materials can be reduced
to obtain the same capacitance as with
. Therefore, such materials are used to increase the capacitance
where the thickness of the materials can be reduced
to obtain the same capacitance as with
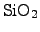 .
.
However, capacitive coupling is often considered as a parasitic effect, for
instance if an array of interconnect lines is considered, where each of them is
used to transmit a different signal.
Capacitive coupling means in this context that an electric signal in one of
these lines influences an electric signal in the other lines. This is refered
to as cross talking. If bus structures for data or addresses are considered too
much cross talk might result in logic device failures.
Hence, for these devices a reduction of the coupling is required. Materials
with lower
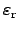 values (
low-
values (
low- materials) offer an alternative to reduce the
capacitive coupling.
materials) offer an alternative to reduce the
capacitive coupling.
Table:
Typical relative dielectric constants for various
high- materials.
materials.
| Material |
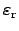
|
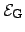
|
References |
| |
[1] |
eV |
|
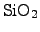
|

|
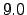
|
[182,183,184] |
| |

|
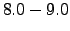
|
[185,186,25] |
| |
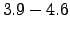 (nitridation) (nitridation)
|
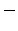
|
[127,187,188] |
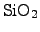 (TEOS)
(TEOS) |

|
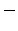
|
[21] |
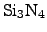
|
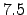
|
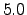
|
[184,189] |
| |

|
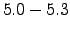
|
[79,185,182,183] |
| |
 (Si-rich) (Si-rich)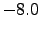 (N-rich) (N-rich)
|
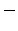
|
[25,79] |
| SiCN |
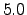
|
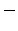
|
[20] |
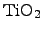
|
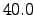
|

|
[189,184] |
| |
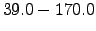
|
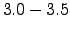
|
[190,182,186] |
|
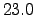
|
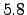
|
[183] |
| |
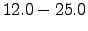
|
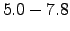
|
[182,184,190,185] |

|
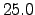
|
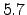
|
[184,185] |
| |
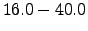
|
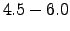
|
[182,183,190] |
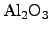
|
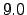
|
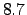
|
[185] |
| |
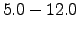
|
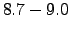
|
[190,182,25] |
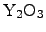
|
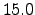
|

|
[185,183] |
| |
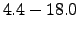
|

|
[186,190] |

|
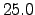
|
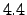
|
[189,184] |
| |
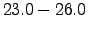
|

|
[190,185,182,183] |
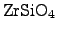
|
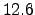
|

|
[190] |
| |
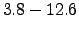
|
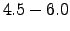
|
[186,183,182] |
A major drawback of all
high- and
low-
and
low- materials is their mechanical
weakness and the complicated and expensive fabrication.
The high
materials is their mechanical
weakness and the complicated and expensive fabrication.
The high
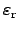 value of
high-
value of
high- materials vanishes at a certain temperature,
where a phase change takes place, reducing
materials vanishes at a certain temperature,
where a phase change takes place, reducing
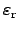 to 1.
This
temperature is called CURIE temperature in analogy to thermo-magnetic effects.
Hence, the thermal budget for these materials is very limited.
The
low-
to 1.
This
temperature is called CURIE temperature in analogy to thermo-magnetic effects.
Hence, the thermal budget for these materials is very limited.
The
low- materials are either porous and very hard or soft, where both
properties are extrema for the fabrication.
For instance, if a soft interlayer dielectric material is processed by a
chemical mechanical polishing (CMP) process step in Cu technology, the
abrasion of the new materials is considerable higher than that of Cu and
materials are either porous and very hard or soft, where both
properties are extrema for the fabrication.
For instance, if a soft interlayer dielectric material is processed by a
chemical mechanical polishing (CMP) process step in Cu technology, the
abrasion of the new materials is considerable higher than that of Cu and
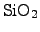 .
The mechanical weakness of this soft material requires additional protective
layers for a normalized CMP procedure. Because many of the
low-
.
The mechanical weakness of this soft material requires additional protective
layers for a normalized CMP procedure. Because many of the
low- materials are
compounds of severals material, they also limit the thermal budget for the
fabrication processes. Otherwise the amorphous and porous materials re-anneal
and the advantageous properties vanish.
materials are
compounds of severals material, they also limit the thermal budget for the
fabrication processes. Otherwise the amorphous and porous materials re-anneal
and the advantageous properties vanish.
Table:
Typical relative dielectric constants for various
low- materials.
materials.
| Material |
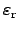
|
References |
| |
[1] |
|
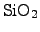
|

|
[182,183,184,185,186,25] |

|
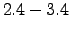
|
[150,148,20,149] |
porous

|
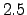
|
[150] |
| SiOCH |
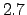
|
[152] |
| organo-silcate glasses (OSGs) |
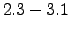
|
[21] |
| aromatic polymers |
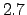
|
[21] |
| with air gaps |
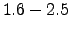
|
[154,155,156] |
Stefan Holzer
2007-11-19
![]() describes how the
electric field
describes how the
electric field
![]() is related to the electric flux density
is related to the electric flux density
![]() ,
,
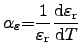
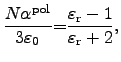
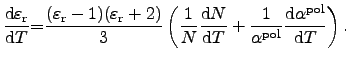
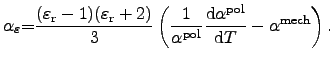
![]() materials, which have a larger
materials, which have a larger
![]() than
than
![]() , and
low-
, and
low-![]() materials, which have a lower
materials, which have a lower
![]() number.
Materials with higher relative permittivities than
number.
Materials with higher relative permittivities than
![]() have a higher
dielectric displacement field (electric flux density) as
have a higher
dielectric displacement field (electric flux density) as
![]() if the same
electric field is applied. Hence, the capacitive
coupling between the two opposite sides of the materials is much tighter as with
if the same
electric field is applied. Hence, the capacitive
coupling between the two opposite sides of the materials is much tighter as with
![]() . Therefore, such materials are used to increase the capacitance
where the thickness of the materials can be reduced
to obtain the same capacitance as with
. Therefore, such materials are used to increase the capacitance
where the thickness of the materials can be reduced
to obtain the same capacitance as with
![]() .
.
![]() values (
low-
values (
low-![]() materials) offer an alternative to reduce the
capacitive coupling.
materials) offer an alternative to reduce the
capacitive coupling.