2.1.1 Seebeck Effect
Figure 2.1:
Thermocouple made of two metal rods.
|
![\includegraphics[width=10cm]{figures/thermocouple.eps}](img159.png) |
Named after his discoverer, the Seebeck effect describes the occurrence of an
electrical voltage induced by a temperature gradient. While the theoretical
interpretation in Seebeck's pioneering paper [1] is surpassed
by his general discovery by far, he also gave an overview of several material
combinations usable in thermocouples as illustrated in
Fig. 2.1.
Two rods of different materials are soldered together and the soldered points
are held at the temperatures
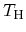 and
and
 , respectively maintaining a
temperature difference
, respectively maintaining a
temperature difference
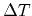 and thus an according temperature gradient
along the rods. On device level, the given temperature difference causes a
certain voltage measured at the device's contacts
and thus an according temperature gradient
along the rods. On device level, the given temperature difference causes a
certain voltage measured at the device's contacts
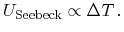 |
(2.1) |
In contrast to the frequently occurring idea in literature, that the Seebeck
effect is based on the temperature dependence of the contact potential, the
reason has to be looked for inside the device. Having a closer look on the
microscopic description, the definition of the Seebeck coefficient is
obtained by approaching infinitesimal small temperature differences. Then, a
local potential gradient is caused by an according temperature gradient, which
are connected by the temperature dependent Seebeck coefficient. Thus, the
definition of the Seebeck coefficient reads
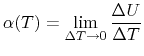 |
(2.2) |
The total voltage measured at the ends of one rod is given by the path integral
along the rod as
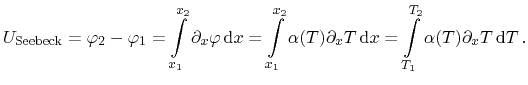 |
(2.3) |
For the entire device, the path integral around the rods has to be evaluated.
Beside the two constitutions of the single rods, the according contact
potentials at the soldered points have to be added. However, the contact
potentials cancel out each other and thus the voltage is given by
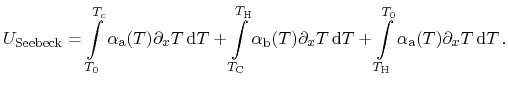 |
(2.4) |
By averaging the temperature dependent Seebeck coefficients along the rods,
a combined coefficient for the material couple under given thermal conditions
can be given as the difference of the single constituents of each rod
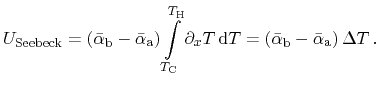 |
(2.5) |
Normally, two materials with Seebeck coefficients of different signs are
chosen in order to gain an accordingly large voltage.
While the Seebeck coefficient of most metals is in the range of
 -
-
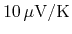 , values of
, values of
 and more are obtained with
semiconductors. Both metals with positive and negative Seebeck coefficients
exist. The choice of according material combinations depends on the intention
of use. For example in measurement applications, high total Seebeck
coefficients are less important than a linear behavior in the desired
temperature range. In semiconductors, the Seebeck coefficient can be varied
by appropriate doping. While n-type semiconductors have negative Seebeck
coefficients, the ones of p-type materials are positive. Quantitative values
obtained in semiconductors can be obtained by analysis of carrier transport.
Based on Boltzmann's equation, expressions for the coefficients are derived
throughout Chapter 3.
and more are obtained with
semiconductors. Both metals with positive and negative Seebeck coefficients
exist. The choice of according material combinations depends on the intention
of use. For example in measurement applications, high total Seebeck
coefficients are less important than a linear behavior in the desired
temperature range. In semiconductors, the Seebeck coefficient can be varied
by appropriate doping. While n-type semiconductors have negative Seebeck
coefficients, the ones of p-type materials are positive. Quantitative values
obtained in semiconductors can be obtained by analysis of carrier transport.
Based on Boltzmann's equation, expressions for the coefficients are derived
throughout Chapter 3.
M. Wagner: Simulation of Thermoelectric Devices
![]() and
and
![]() , respectively maintaining a
temperature difference
, respectively maintaining a
temperature difference
![]() and thus an according temperature gradient
along the rods. On device level, the given temperature difference causes a
certain voltage measured at the device's contacts
and thus an according temperature gradient
along the rods. On device level, the given temperature difference causes a
certain voltage measured at the device's contacts
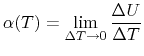
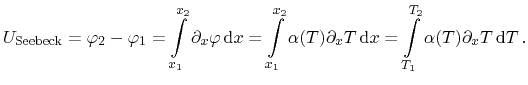
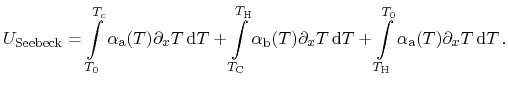
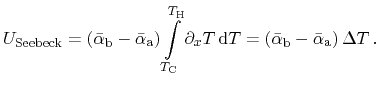
![]() -
-
![]() , values of
, values of
![]() and more are obtained with
semiconductors. Both metals with positive and negative Seebeck coefficients
exist. The choice of according material combinations depends on the intention
of use. For example in measurement applications, high total Seebeck
coefficients are less important than a linear behavior in the desired
temperature range. In semiconductors, the Seebeck coefficient can be varied
by appropriate doping. While n-type semiconductors have negative Seebeck
coefficients, the ones of p-type materials are positive. Quantitative values
obtained in semiconductors can be obtained by analysis of carrier transport.
Based on Boltzmann's equation, expressions for the coefficients are derived
throughout Chapter 3.
and more are obtained with
semiconductors. Both metals with positive and negative Seebeck coefficients
exist. The choice of according material combinations depends on the intention
of use. For example in measurement applications, high total Seebeck
coefficients are less important than a linear behavior in the desired
temperature range. In semiconductors, the Seebeck coefficient can be varied
by appropriate doping. While n-type semiconductors have negative Seebeck
coefficients, the ones of p-type materials are positive. Quantitative values
obtained in semiconductors can be obtained by analysis of carrier transport.
Based on Boltzmann's equation, expressions for the coefficients are derived
throughout Chapter 3.