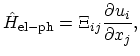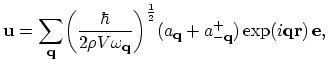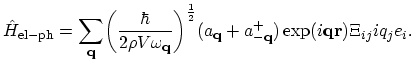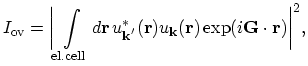From the quantum theory point of view instead of waves (2.98) phonons are introduced through the second quantization formalism
(see Appendix A). Phonons represent quasi-particles moving in the crystal. The energy of a phonon is
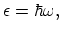 |
(2.99) |
where 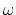 is the frequency of the classical wave (2.98). The wave vector
is the frequency of the classical wave (2.98). The wave vector 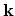 in (2.98) determines a phonon quasi-momentum as
follows:
in (2.98) determines a phonon quasi-momentum as
follows:
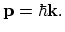 |
(2.100) |
This quantity is not unique as any quasi-momentum
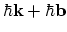 , where
, where 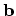 is a reciprocal lattice vector, is physically equivalent
to
is a reciprocal lattice vector, is physically equivalent
to
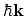 . The velocity of a phonon is determined as the group velocity of the corresponding classical waves
. The velocity of a phonon is determined as the group velocity of the corresponding classical waves
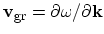 and has the form:
and has the form:
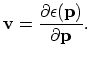 |
(2.101) |
All the properties of the classical wave spectrum are valid for the energy spectrum of phonons. In particular it has 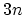 branches, three of which are
acoustic ones. The wave density is now interpreted as the phonon density of states.
branches, three of which are
acoustic ones. The wave density is now interpreted as the phonon density of states.
The free wave motion is considered as the free motion of non-interacting phonons. Inclusion of the anharmonicity leads to scattering processes in the
phonon gas. These scattering processes restore the thermal equilibrium of the phonon gas. The processes conserve the quasi-momentum.
However, this is only valid within an addition of a reciprocal lattice vector
 .
.
It should be noted that the phonon concept appears only as the quantum mechanical description of the collective atomic motion in a crystal and that
phonons cannot be identified with individual atoms.
As applied to the electron transport in semiconductors the interaction between the electron gas and the phonon gas plays an important
role. In this work only covalent semiconductors are considered. In this case the electron-phonon interaction can be successfully described using the
deformation-potential approach [25]. In this case the interaction Hamiltonian in (2.95) is given as:
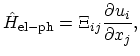 |
(2.102) |
where 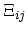 is the deformation-potential tensor describing the shift of a band per unit deformation. Using the continuous medium approximation the
ion displacement takes the form:
is the deformation-potential tensor describing the shift of a band per unit deformation. Using the continuous medium approximation the
ion displacement takes the form:
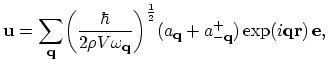 |
(2.103) |
where  is the density of the crystal and
is the density of the crystal and  and
and 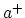 stand for the phonon annihilation and creation operators, respectively. Therefore the
interaction Hamiltonian is:
stand for the phonon annihilation and creation operators, respectively. Therefore the
interaction Hamiltonian is:
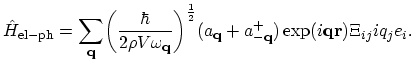 |
(2.104) |
It is convenient to interpret this equation in terms of phonon emission and absorption processes. If in (2.95) 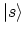 stands for
stands for
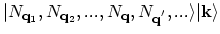 then in the sum over
then in the sum over  only two
terms will contribute: one from
only two
terms will contribute: one from
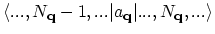 with pre-factor
with pre-factor
 and the second from
and the second from
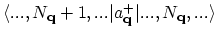 with pre-factor
with pre-factor
 . Thus using (2.95) the phonon
differential scattering rates in the kinetic equation (2.60) take the form:
. Thus using (2.95) the phonon
differential scattering rates in the kinetic equation (2.60) take the form:
| |
|
![$\displaystyle S^\mathrm{ab}(\vec{k},\vec{k}^{'},\vec{r},t)=\frac{N_{\vec{q}}I_\...
...vec{q}}}
\delta[\epsilon(\vec{k}^{'})-\epsilon(\vec{k})-\hbar\omega_{\vec{q}}],$](img437.png) |
|
| |
|
![$\displaystyle S^\mathrm{em}(\vec{k},\vec{k}^{'},\vec{r},t)=\frac{(N_{\vec{q}}+1...
...vec{q}}}
\delta[\epsilon(\vec{k}^{'})-\epsilon(\vec{k})+\hbar\omega_{\vec{q}}],$](img438.png) |
(2.105) |
where
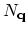 is given by (2.34), that is, within this work the phonon gas is assumed to be in the equilibrium which is not valid in general
[26,27,28].
is given by (2.34), that is, within this work the phonon gas is assumed to be in the equilibrium which is not valid in general
[26,27,28].
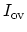 is the overlap integral:
is the overlap integral:
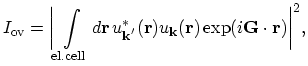 |
(2.106) |
where 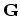 is a reciprocal lattice vector. For intervalley transitions the angle between initial and final states depends
mainly on the valleys involved in the transition,
is a reciprocal lattice vector. For intervalley transitions the angle between initial and final states depends
mainly on the valleys involved in the transition,
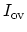 is nearly constant [29] and can be taken into account by renormalizing the
corresponding coupling constant.
is nearly constant [29] and can be taken into account by renormalizing the
corresponding coupling constant.
The most important phonon scattering processes for covalent semiconductors can be described by (2.105). Those of them which are
important for Si, Ge and SiGe are briefly given below.
S. Smirnov:
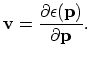
![]() .
.