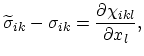When a solid is strained by external forces the positions between atoms change and it is in a non-equilibrium state. As a result some internal forces
appear. These forces tend to
return the strained solid to its equilibrium state. The induced forces are called the internal stresses. If a solid is not strained, the internal stresses
vanish.
Considering some volume of a solid the total force applied to it can be written as an integral over the same volume:
 |
(3.21) |
where 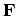 is the force applied to a unit volume of the solid. As the forces between internal parts of the volume are balanced, only
external parts contribute. Further the macroscopic principle3.2 means that the total force can only be expressed as a surface integral.
is the force applied to a unit volume of the solid. As the forces between internal parts of the volume are balanced, only
external parts contribute. Further the macroscopic principle3.2 means that the total force can only be expressed as a surface integral.
Therefore each of the three components of the total force (3.21) should be a surface integral. This fact implies the that vector 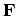 must
be the divergence of a tensor of rank two. Denoting this tensor as
must
be the divergence of a tensor of rank two. Denoting this tensor as
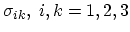 ,
, 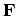 can be expressed as:
can be expressed as:
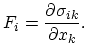 |
(3.22) |
From this expression the following formula is obtained:
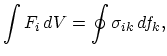 |
(3.23) |
which is only valid for the weak strain condition3.3.
The tensor
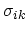 is called the stress tensor. As it can be seen from (3.23),
is called the stress tensor. As it can be seen from (3.23),
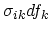 is the
is the 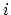 -th component of the
force applied to the surface element
-th component of the
force applied to the surface element 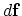 . Choosing a surface element in the
. Choosing a surface element in the 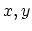 ;
; 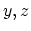 and
and  planes it can be seen that the component
planes it can be seen that the component
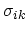 of the stress tensor is the
of the stress tensor is the 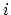 -th component of the force applied to a unit area perpendicular to the
-th component of the force applied to a unit area perpendicular to the 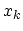 axis. For example, the normal
component of the force applied to a unit area perpendicular to
axis. For example, the normal
component of the force applied to a unit area perpendicular to 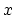 is equal to
is equal to
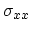 and the forces tangential to it are equal to
and the forces tangential to it are equal to
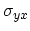 and
and
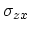 as it is shown in Fig. 3.6.
as it is shown in Fig. 3.6.
Figure 3.6:
The stress tensor components in terms of the applied forces.
|
|
It is well known that the moment of force can be written as an antisymmetric tensor of rank two3.4. Thus the total moment of force applied to the volume under consideration is:
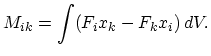 |
(3.24) |
Like the total force the moment of force also must be expressed through a surface integral. Substituting (3.22) into (3.24) gives:
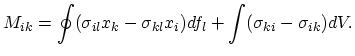 |
(3.25) |
This expression represents a surface integral only if the following equality is satisfied:
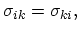 |
(3.26) |
which removes the volume integral in (3.25). Hence an important property of the stress tensor is its symmetry. In principle the
integrand in the volume integral in (3.25) can be a complete divergence of a tensor of rank three which is antisymmetric in the first two
indices. This tensor must be expressed through derivatives like
 . Thus the stress tensor would contain terms of higher
order derivatives of the displacement vector
. Thus the stress tensor would contain terms of higher
order derivatives of the displacement vector 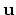 . Within this work such terms are considered negligible. Hence the symmetry of the stress
tensor is again justified.
. Within this work such terms are considered negligible. Hence the symmetry of the stress
tensor is again justified.
It should be noted that the stress tensor can be transformed into diagonal form even without this approximation. The point is that its definition
(3.22) is not unique. Any transformation
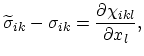 |
(3.27) |
where the tensor
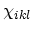 is antisymmetric with respect to the last two indices, does not change the force
is antisymmetric with respect to the last two indices, does not change the force 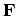 .
S. Smirnov:
.
S. Smirnov:
![]() must
be the divergence of a tensor of rank two. Denoting this tensor as
must
be the divergence of a tensor of rank two. Denoting this tensor as
![]() ,
, ![]() can be expressed as:
can be expressed as:
![]() is called the stress tensor. As it can be seen from (3.23),
is called the stress tensor. As it can be seen from (3.23),
![]() is the
is the ![]() -th component of the
force applied to the surface element
-th component of the
force applied to the surface element ![]() . Choosing a surface element in the
. Choosing a surface element in the ![]() ;
; ![]() and
and ![]() planes it can be seen that the component
planes it can be seen that the component
![]() of the stress tensor is the
of the stress tensor is the ![]() -th component of the force applied to a unit area perpendicular to the
-th component of the force applied to a unit area perpendicular to the ![]() axis. For example, the normal
component of the force applied to a unit area perpendicular to
axis. For example, the normal
component of the force applied to a unit area perpendicular to ![]() is equal to
is equal to
![]() and the forces tangential to it are equal to
and the forces tangential to it are equal to
![]() and
and
![]() as it is shown in Fig. 3.6.
as it is shown in Fig. 3.6.