previous up next Dissertation Enzo Ungersboeck contents
 Previous: 3.7.1 Effective Electron Mass in Unstrained Si   Up: 3.7 The kp method   Next: Energy Dispersion of the Conduction Band Minimum of


3.7.2 Strain Effect on the Si Conduction Band Minimum

Having discussed strain-induced shifts of the conduction bands in Section 3.6.1, here the effect of strain on the effective electron mass in the lowest conduction band is analyzed.

Equation (3.47) neglects the lifting of the degeneracy of the lowest two conduction bands induced by shear strain and describes the energy shift of the conduction bands as a whole. Apart from the direction of the wavevector $ \mathitbf{k}$ indicating the location of the valley no information is required in (3.47) to determine the shift of the valley minima. As a consequence, the shift of a valley is independent of the exact value of the wavevector $ \mathitbf{k}$, and all $ \mathitbf{k}$ points belonging to a particular valley experience the same shift. As the effective mass relates to the curvature of the energy band, which is not changed by an overall shift in energy, equation (3.47) implies that the effective electron mass is not affected by strain.

There is clear experimental evidence that shear strain changes the effective masses of electrons in the lowest conduction band [Hensel65] and also the exciton spectrum of Si [Laude71]. In order to explain these experiments, the splitting of the two lowest conduction bands at the $ X$ symmetry point induced by shear strain (see Section 3.6.2) has to be taken into account. From (3.52) the lifting of the degeneracy at the $ X$ point can be calculated using the deformation potential constant $ \Xi _{u'}$. However, since (3.52) is only valid for the symmetry point $ X$, it cannot be used to predict the effect of strain on the valley minima $ {\ensuremath{\mathitbf{k}}}_{\mathrm{min}}$. To determine the change of the effective electron mass under shear strain, a degenerate kp theory around the symmetry point $ X$ must be applied, since the two conduction bands $ \Delta _1$ and $ \Delta _{2'}$ are degenerate in the unstrained lattice.

Using the theory of invariants [Luttinger56] Bir and Pikus [Bir74] determined a suitable choice of matrices describing the Hamiltonian at the points $ X=\frac{2\pi}{a_0} (0,0,\pm 1)$

$\displaystyle \mathcal{H}(\hat{{\ensuremath{\varepsilon_{}}}},{\ensuremath{\mat... ...th{\varepsilon_{xy}}}) + A_4 \ensuremath{{\underaccent{\bar}{\sigma}}}_z k_z\ .$ (3.67)

Here,

$\displaystyle \lambda = A_1k_z^2 + A_2 (k_x^2 + k_y^2) + D_1{\ensuremath{\varep... ...}} + D_2 ({\ensuremath{\varepsilon_{xx}}} + {\ensuremath{\varepsilon_{yy}}})\ ,$ (3.68)

$ A_1$ to $ A_2$ are scalar constants and $ \ensuremath{{\underaccent{\bar}{\sigma}}}_x$ and $ \ensuremath{{\underaccent{\bar}{\sigma}}}_z$ denote the spinor matrices given by

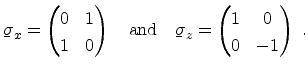$\displaystyle \ensuremath{{\underaccent{\bar}{\sigma}}}_x = \begin{pmatrix}0 & ... ...nderaccent{\bar}{\sigma}}}_z = \begin{pmatrix}1 & 0\\ 0 & -1\\ \end{pmatrix}\ .$ (3.69)

The scalars $ D_1, D_2$, and $ D_2$ are related to the deformation potential constants $ \Xi_u, \Xi_d$, and $ \Xi _{u'}$ via

$\displaystyle D_1$ $\displaystyle = \Xi_u + \Xi_d\ ,$ (3.70)
$\displaystyle D_2$ $\displaystyle = \Xi_d\ ,$ (3.71)
$\displaystyle D_3$ $\displaystyle = 2 \Xi_{u'}\ .$ (3.72)

The energy dispersion of the first and the second conduction band can be determined as the eigenvalues of (3.67)

$\displaystyle E_{\pm}(\hat{{\ensuremath{\varepsilon_{}}}},{\ensuremath{\mathitb... ...qrt{A_4^2k_z^2 + (2\Xi_{u'} {\ensuremath{\varepsilon_{xy}}} + A_3k_x k_y)^2}\ ,$ (3.73)

where $ E_{-}$ describes the dispersion of $ \Delta _1$ and $ E_{+}$ that of $ \Delta _{2'}$.

Assuming that this expansion around the $ X$ point is valid up to the minimum of the lowest conduction band at $ {\ensuremath{\mathitbf{k}}}_{\mathrm{min}} = \frac{2\pi}{a_0} (0,0,\pm0.85)$, the constant $ A_4$ can be related to $ A_1$

$\displaystyle 0 = \frac{\partial E_{-}(\hat{{\ensuremath{\varepsilon_{}}}}=0,{\... ...hitbf{k}}}_{\mathrm{min}}} = 2 A_1 k_0 + \frac{A_4^2k_0}{\sqrt{A_4^2 k_0^2}}\ .$ (3.74)

Here, $ k_0 = 0.15\frac{2\pi}{a_0}$ denotes the distance of the conduction band minimum of unstrained Si from the $ X$ point. From (3.74) the magnitude of $ A_4$ can be determined

$\displaystyle \vert A_4\vert = 2 A_1 k_0\ .$ (3.75)

In the following the effect of shear strain on the shape of the lowest conduction band is derived by two different methods.


Subsections


previous up next   contents
 Previous: 3.7.1 Effective Electron Mass in Unstrained Si   Up: 3.7 The kp method   Next: Energy Dispersion of the Conduction Band Minimum of
E. Ungersboeck: Advanced Modelling Aspects of Modern Strained CMOS Technology