 |
|
||||
BiographyJosef Weinbub is an Associate Professor of High Performance Simulation in Micro- and Nanoelectronics, an IEEE Senior Member, and the Co-Chair of the IEEE Nanotechnology Council's Modeling and Simulation Technical Committee. He obtained the doctoral degree in Computational Microelectronics and the venia docendi (habilitation) in the field of Micro- and Nanoelectronics from the TU Wien. He was a visiting researcher at the EPCC, University of Edinburgh and at the Device Modelling Group, University of Glasgow, Scotland, UK as well as at SILVACO Inc., Santa Clara, CA, USA. He founded and now chairs the master’s program Computational Science and Engineering at the TU Wien and is involved with several international scientific conferences in various management and scientific roles. He is an Associate Editor of the Journal of Computational Electronics and a Principal Investigator of various research projects funded by, e.g., the Austrian Science Fund and the Christian Doppler Research Association. Together with his team he investigates cutting-edge research problems in the area of computational micro- and nanoelectronics. Research Interests:Josef’s research interests are focused on developing and utilizing computationally highly demanding simulations in process TCAD and electron quantum transport to study cutting edge challenges in semiconductor fabrication and next generation electron-based quantum devices and systems. Regarding process TCAD, he particularly focuses on parallel algorithm and data structure research with a particular focus on accelerating flux calculations. With respect to electron quantum transport, he focuses on advancing a time-dependent particle Wigner simulation methodology. This is particularly relevant for the field of electron quantum optics, which studies electron-based quantum optics principles (e.g., interference of electron-wave) by being able to control individual electrons. He applies the developed methods by studying dynamic single electron and entangled double electron systems under the influence of electromagnetic fields, and thereby advances the understanding of electromagnetic electron control, coherent electron sources and waveguides, as well as interferometers. Software Development:Particle Wigner Simulator: ViennaWD Research Topics:High Performance Computing Past Public Projects:CDG - Christian Doppler Laboratory for High Performance TCAD FWF - Wigner Transport Dynamics of Spatial Electron Entanglement EU - European Master For High Performance Computing FWF - General Nano-Electromagnetic Quantum Phase Space Model |
|||||
Controlling Single Electrons by Non-Uniform Magnetic Fields
Controlling single electrons is among the core mechanisms in electron quantum optics. There, electron control allows for the specific guidance and influence of the evolution of individual electrons in transport channels and interaction systems, e.g., interferometers. Fundamental are mechanisms based on electromagnetic fields, providing additional degrees of freedom for electron control. However, minimal experience and understanding of the intricate interplay between electromagnetic fields and the involved single-electron quantum transport is available. We introduce a recently advanced Wigner theory incorporating non-uniform magnetic fields into the quantum transport model. We show simulation studies covering magnetotunneling and snake states, where the influence of the electromagnetic fields manipulates electron evolution. We consider 2D transport in the xy-plane and a non-uniform magnetic field in the direction normal to the plane B=(0,0,B(y)), where B(y)=B0+B1 y. Non-interacting electrons are periodically injected (minimum uncertainty Wigner states) at the bottom and center of the simulation domain. Concerning magnetotunneling, the electrons evolve in the +y direction and collide with a 0.3 eV and 1 nm potential barrier. We consider different cases for (B0,B1): (0,0), (-6T,0), and (-6T,0.2 T⁄nm), cf., simulated electron densities in Fig. 1-3. As can be seen, the electron can, in principle, tunnel into the upper half of the domain. The mean densities follow the classical paths (indicated lines) per the Ehrenfest theorem. Fig. 1 shows a fine oscillatory structure beyond the barrier, which, however, is destroyed by the electron-bending magnetic field in the case shown in Fig. 2. In Fig. 3, the magnetic field changes its direction after the barrier, giving rise to a snake type of evolution. In addition, a fine oscillatory structure beyond the barrier manifests, like the case shown in Fig. 1. This behavior is linked to the magnetic field being zero around the barrier and a local interplay between the magnetic field and the electric field associated with the barrier.
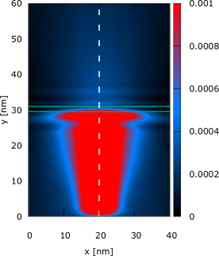
Fig. 1: Magnetotunneling electron density for (B_0,B_1 )=(0,0).
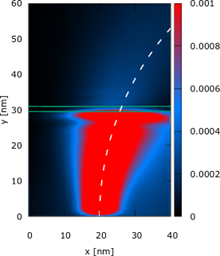
Fig. 2: Magnetotunneling electron density for (B_0,B_1 )=(-6T,0).

Fig. 3: Magnetotunneling electron density for (B_0,B_1 )=(-6T,0.2 T⁄nm).


