|
|
|
|
||
| Previous: 3. Strained Bulk Band Structure Up: 3. Strained Bulk Band Structure Next: 3.2 Stress | ||||
In this section the basic expressions and notations for strain in cubic
crystalline solids are established. A more detailed analysis can be found in
textbooks [Bir74,Kittel96,Singh93]. Starting point for the definition of
strain in a system is a set of orthonormal vectors
![]() and
and
![]() embedded in an unstrained solid. These vectors are distorted to
embedded in an unstrained solid. These vectors are distorted to
![]() , and
, and
![]() under the influence of a uniform
deformation
under the influence of a uniform
deformation
| (3.1) |
For a uniform deformation of a body point originally located at
![]() the displacement to
the displacement to
![]() is defined as
is defined as
| (3.2) |
| (3.3) |
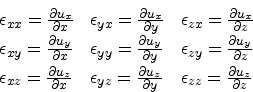 |
(3.4) |
Thus, the displacements can be written as
![]() .
.
Usually, instead of the displacements
![]() , the strain tensor
, the strain tensor
![]() is used to quantify the the deformation of an body in three
dimensions. In the case of small deformations, the strain tensor is known as
the Green tensor or Cauchy's infinitesimal strain tensor, which
is defined as
is used to quantify the the deformation of an body in three
dimensions. In the case of small deformations, the strain tensor is known as
the Green tensor or Cauchy's infinitesimal strain tensor, which
is defined as
Frequently, in literature the engineering strains ![]() are used which are related to
the components of the strain tensor via
are used which are related to
the components of the strain tensor via
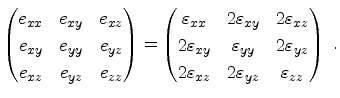 |
(3.6) |
|
|
|
|
||
| Previous: 3. Strained Bulk Band Structure Up: 3. Strained Bulk Band Structure Next: 3.2 Stress | ||||