


Next: Bibliography
Up: Dissertation Siddhartha Dhar
Previous: Appendix A
Appendix B
From (4.118) and (4.119) we get
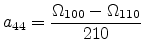 |
(6.10) |
After elimination of  , the system of equations (4.118)
to (4.122) can be expressed in matrix form as
, the system of equations (4.118)
to (4.122) can be expressed in matrix form as
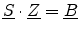 |
(6.11) |
where
To determine the four coefficients from the overdetermined system (6.11),
we solve (4.118) to (4.120) exactly and minimize the error in
(4.121) and (4.122). Since
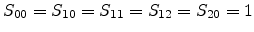 , using the new variables the equations can be written as
, using the new variables the equations can be written as
Next, we eliminate 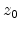 by subtracting (6.15) from
(6.14), (6.16), and (6.17).
by subtracting (6.15) from
(6.14), (6.16), and (6.17).
To eliminate 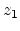 , (6.18) is multiplied by
, (6.18) is multiplied by
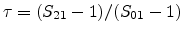 to obtain
to obtain
Subtracting (6.21) from (6.19) and (6.20)
gives
with
The least square minimum can be obtained by setting the derivative to zero,
which gives
With the definitions (6.24) to (6.26) and
using (6.12) we get
Substituting back the value of 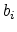 's from (6.13)
into (6.29) to (6.31) gives
equations (4.123)-(4.126).
's from (6.13)
into (6.29) to (6.31) gives
equations (4.123)-(4.126).



Next: Bibliography
Up: Dissertation Siddhartha Dhar
Previous: Appendix A
S. Dhar: Analytical Mobility Modeling for Strained Silicon-Based Devices
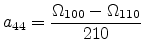
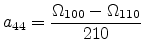
![]() , the system of equations (4.118)
to (4.122) can be expressed in matrix form as
, the system of equations (4.118)
to (4.122) can be expressed in matrix form as