4.4 Body Contact

The order of magnitude of the involved currents can be estimated by looking at simulations of the same device, but with a body contact attached. This body contact prevents the body potential from dropping below its equilibrium value. Fig. 4.6 shows the output characteristics of this device. Because of the pinned body potential the drain current is not much affected by impact-ionization.

Figure 4.6: Output characteristics of the SOI with a body contact (Device 2) obtained by energy transport simulations.

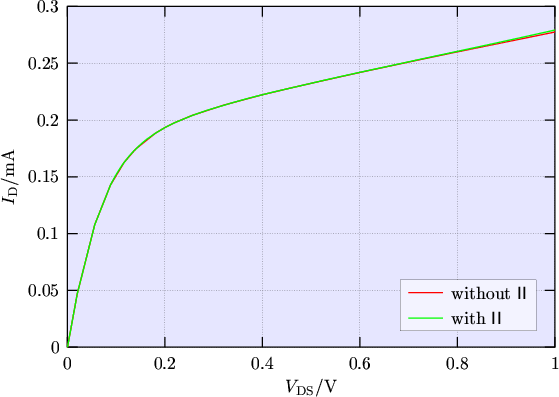The kink in the drain current does not appear because both contributing effects are suppressed, namely the body effect and the amplification of the impact-ionization current through the bipolar effect. As expected, a positive output conductance is obtained.

The strong influence of impact-ionization can be seen in the corresponding bulk currents (Fig. 4.7). With impact-ionization included the expected result of a body current flowing out of the transistor is obtained ( $I_\mathrm{B} < 0$). But if in contrast impact-ionization is neglected there is a body current flowing into the device ( $I_\mathrm{B} > 0$), which is several orders of magnitude smaller. It is to note that the real substrate current due to impact-ionization has the opposite sign. The situation of a positive substrate current shows that even in this bulk MOSFET hot electron diffusion into the p-body occurs. Note that this is a prediction of the energy transport model only, and is not confirmed by experimental data.

Figure 4.7: Bulk currents of the SOI with body contact (Device 2) obtained by energy transport simulations.

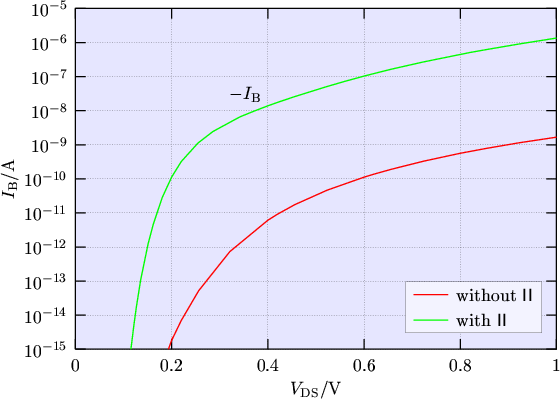To estimate if the resulting drain current obtained by the drift-diffusion simulation using impact-ionization shown in Fig. 4.2 is really caused by the increased body potential, simulations using the same transistor but with a body contact applied (Device 2) were made. The results are shown in Fig. 4.8 where the curve from Fig. 4.2 which used impact-ionization is depicted again--this time the full $I_\mathrm{D}$ range is shown.

Figure 4.8: Comparison of the drain currents of the SOI (Device 1) and the device with body contact (Device 2) obtained by drift-diffusion simulations.

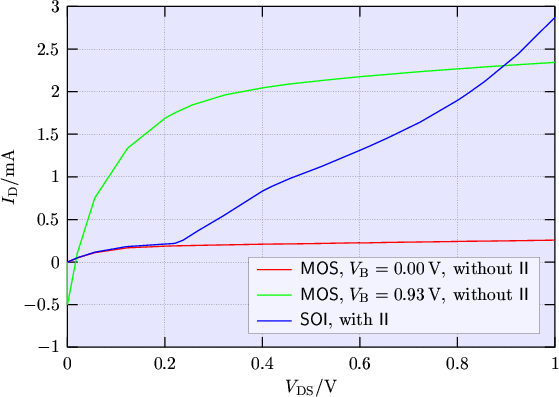From Fig. 4.3 it can be seen that the body potential is shifted from $- 0.46 \, \mathrm{V}$ at $V_\mathrm{DS} = 0.0 \, \mathrm{V}$ to $+ 0.47 \, \mathrm{V}$ at $V_\mathrm{DS} = 1.0 \, \mathrm{V}$ resulting in a total shift of $0.93 \, \mathrm{V}$. This voltage is now applied at the body contact of Device 2. In this case the source-body diode (and at small $V_\mathrm{DS}$ even the drain-body diode) is biased in forward direction yielding a negative drain current of $I_\mathrm{D} = - 0.5 \, \mathrm{mA}$ at $V_\mathrm{DS} = 0 \, \mathrm{V}$. Accounting for this negative current offset total agreement with the curve using impact-ionization is obtained at $V_\mathrm{DS} = 1 \, \mathrm{V}$.

M. Gritsch: Numerical Modeling of Silicon-on-Insulator MOSFETs PDF