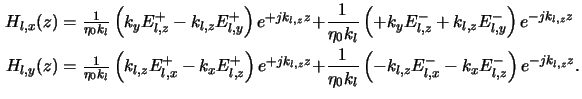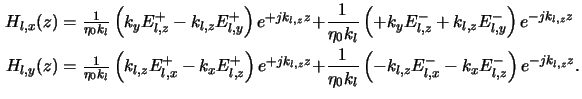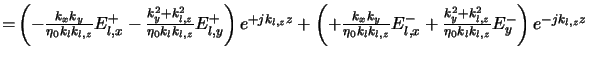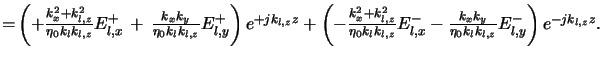


Next: Matrix Notation
Up: C.1 One Homogeneous Planar
Previous: Electric Field
With the help of the orthogonality requirement
Hl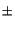 = 1/(
= 1/(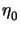 kl) k
kl) k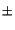 l x E
l x E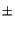 l
the magnetic field phasors
Hl+ and
Hl- are easily
calculated from the electric ones. From (C.1) we obtain similar
to (C.5) for the
z-dependent part of the lateral magnetic field components
The vertical amplitudes
E
l
the magnetic field phasors
Hl+ and
Hl- are easily
calculated from the electric ones. From (C.1) we obtain similar
to (C.5) for the
z-dependent part of the lateral magnetic field components
The vertical amplitudes
E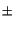 l, z can readily be eliminated with the help of
(C.4) resulting in
l, z can readily be eliminated with the help of
(C.4) resulting in
Heinrich Kirchauer, Institute for Microelectronics, TU Vienna
1998-04-17