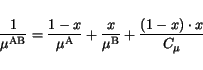


Next: 3.5 Velocity Saturation
Up: 3.4 Carrier Mobility
Previous: 3.4.4 High-Field Mobility for
Subsections
The deviation from the ohmic low-field mobility is modeled as a function of the
carrier temperature, 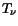 , after Hänsch [180].
, after Hänsch [180].
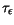 denotes the energy relaxation times
and
denotes the energy relaxation times
and
 are the saturation velocities calculated respectively in
(3.138) and in (3.134).
are the saturation velocities calculated respectively in
(3.138) and in (3.134).
For some III-V semiconductor materials a multi-valley mobility model can be
used. In the following model a weighted mean is calculated from the low-field
mobilities of the 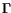 - and
- and 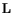 -electrons [181].
-electrons [181].
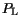 denotes the ratio of the
denotes the ratio of the 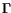 and
and 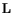 -valley populations
[86]. The valley mobilities
-valley populations
[86]. The valley mobilities
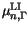 and
and
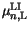 account for impurity scattering after
(3.97), and are constant with respect to the lattice
temperature.
account for impurity scattering after
(3.97), and are constant with respect to the lattice
temperature.
Table 3.29:
Parameter values for the two-valley HD mobility model
| Parameter |
GaAs |
Unit |
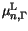 |
8000 |
cm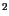 /Vs /Vs |
 |
400 |
cm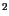 /Vs /Vs |
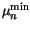 |
1500 |
cm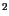 /Vs /Vs |
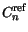 |
1.426e+17 |
cm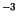 |
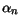 |
0.5385 |
|
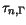 |
1.2 |
ps |
 |
0.6 |
ps |
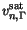 |
2.5e5 |
m/s |
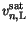 |
0.9e5 |
m/s |
|
In the case of alloy materialsthe model employs the low-field mobilities
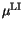 of
the basic materials (A and B) and combines them by a harmonic mean.
of
the basic materials (A and B) and combines them by a harmonic mean.
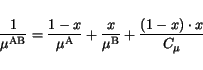 |
(3.133) |
 is referred to as nonlinear or bowing parameter.
is referred to as nonlinear or bowing parameter.
Table 3.30:
Parameter values for mobility model for alloy materials
| Material |
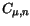 [cm [cm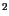 /Vs] /Vs] |
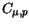 [cm [cm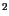 /Vs] /Vs] |
| SiGe |
1e6 |
-100 |
| AlGaAs |
180 |
1e6 |
| InGaAs |
1e6 |
1e6 |
| InAlAs |
1e6 |
1e6 |
| InAsP |
1e6 |
1e6 |
| GaAsP |
1e6 |
1e6 |
| InGaP |
1e6 |
1e6 |
|
For calculation of the high field mobilities no additional parameters need to be
specified for the model. The respective interpolations between the basic materials are carried out in the models for the saturation velocities and, in the case
of HD simulation, of the energy relaxation times.



Next: 3.5 Velocity Saturation
Up: 3.4 Carrier Mobility
Previous: 3.4.4 High-Field Mobility for
Vassil Palankovski
2001-02-28
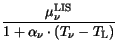
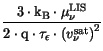
![]() - and
- and ![]() -electrons [181].
-electrons [181].
![]() denotes the ratio of the
denotes the ratio of the ![]() and
and ![]() -valley populations
[86]. The valley mobilities
-valley populations
[86]. The valley mobilities
![]() and
and
![]() account for impurity scattering after
(3.97), and are constant with respect to the lattice
temperature.
account for impurity scattering after
(3.97), and are constant with respect to the lattice
temperature.