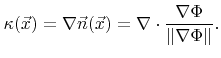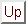


Next: 3.4 Acceleration Techniques
Up: 3.3 Approximations to Geometric
Previous: 3.3.1 Surface Normal
3.3.2 Curvature
Solving the LS equation with a velocity field that is proportional to the curvature can be used for smoothing as will be demonstrated in Section 4.5. For an arbitrary point 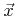 the (mean) curvature of the corresponding LS is defined as
the (mean) curvature of the corresponding LS is defined as
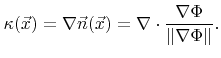 |
(3.24) |
At grid points
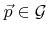 the mean curvature can be approximated by [110]
the mean curvature can be approximated by [110]
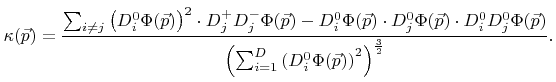 |
(3.25) |



Next: 3.4 Acceleration Techniques
Up: 3.3 Approximations to Geometric
Previous: 3.3.1 Surface Normal
Otmar Ertl: Numerical Methods for Topography Simulation
![]() the (mean) curvature of the corresponding LS is defined as
the (mean) curvature of the corresponding LS is defined as