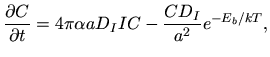In [109] the equation describing interstitial cluster kinetics is
described as
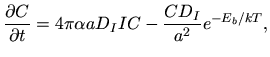 |
(5.17) |
where 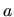 denotes the interatomic spacing,
denotes the interatomic spacing, 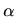 the capture radius expressed
in units of the interatomic spacing,
the capture radius expressed
in units of the interatomic spacing,
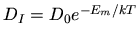 the interstitial
diffusivity,
the interstitial
diffusivity, 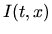 the concentration of unclustered interstitials,
the concentration of unclustered interstitials,  the concentration of clustered interstitials,
the concentration of clustered interstitials, 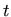 the time,
the time,  the annealing
temperature in Kelvin, and
the annealing
temperature in Kelvin, and 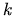 the Boltzmann constant. TSUPREM-IV uses a general
clustering model that uses many of the models proposed in literature as
subsets. The main formula for the change of the concentration of clustered
interstitials is [13]
the Boltzmann constant. TSUPREM-IV uses a general
clustering model that uses many of the models proposed in literature as
subsets. The main formula for the change of the concentration of clustered
interstitials is [13]
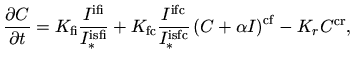 |
(5.18) |
where
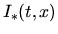 denotes an equilibrium concentration of interstitials. All
other symbols of (5.18) are parameters that need to be
adjusted.
denotes an equilibrium concentration of interstitials. All
other symbols of (5.18) are parameters that need to be
adjusted.
 ,
,
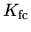 , and
, and 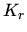 are the reactions
constants which have the form
are the reactions
constants which have the form
with coefficients
 ,
,
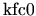 , and
, and
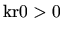 . Since the coefficients are all positive the first two terms of
(5.18) describe the formation of clusters whereas the last term
describes its dissolution. The term
. Since the coefficients are all positive the first two terms of
(5.18) describe the formation of clusters whereas the last term
describes its dissolution. The term
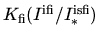 describes the joining of
two clusters5.7. Therefore, the parameter values
describes the joining of
two clusters5.7. Therefore, the parameter values
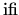 and
and
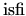 are expected to be
are expected to be 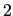 . The second term,
. The second term,
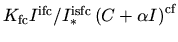 , describes the case where an unclustered interstitial
joins a cluster. A value of
, describes the case where an unclustered interstitial
joins a cluster. A value of 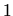 for
for
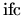 and
and
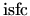 is
assumed.
is
assumed.
2003-03-27