« Previous Up Next »Contents
Previous: 2.3 Gate voltage dependence Top: 2 On the first Component: the Subthreshold Hysteresis Next: 2.5 Crystal face dependence

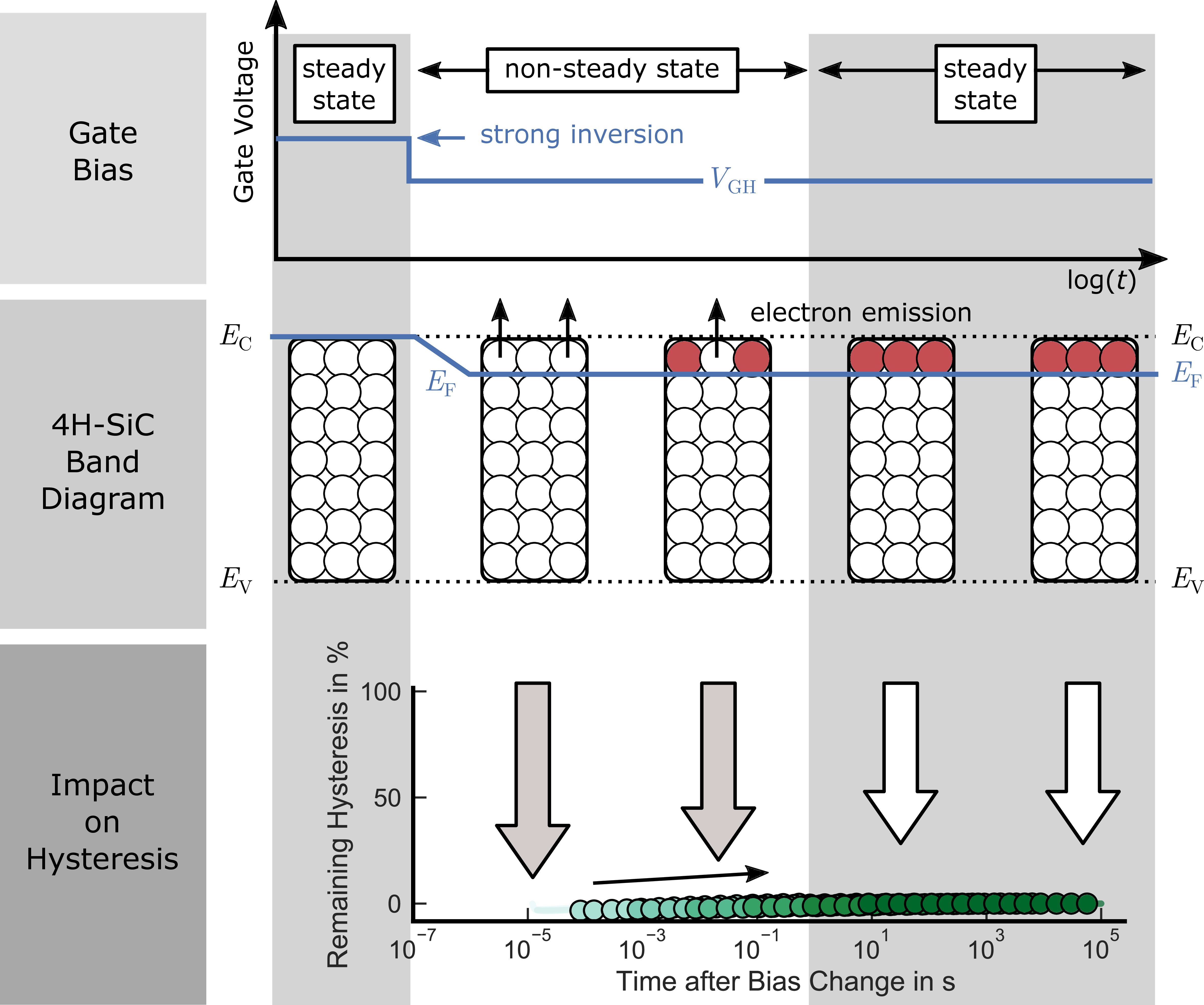
2.4 Time and temperature dependence

As shown in Fig. 2.3, biasing the device in accumulation ( $\ac {vg} = \SI {-15}{\V }$ ) is sufficient to completely charge the interface states and observe the maximum hysteresis. The positive charging of the interface states at this voltage level happens within several nanoseconds [111], and can therefore not be resolved due to the technical limitations of our measurement setup. Due to this, the next sections focus on the time and temperature dependence of the recovery, assuming the interface is always completely positively charged, which will be the case after an accumulation pulse longer than approximately 500 ns [111].

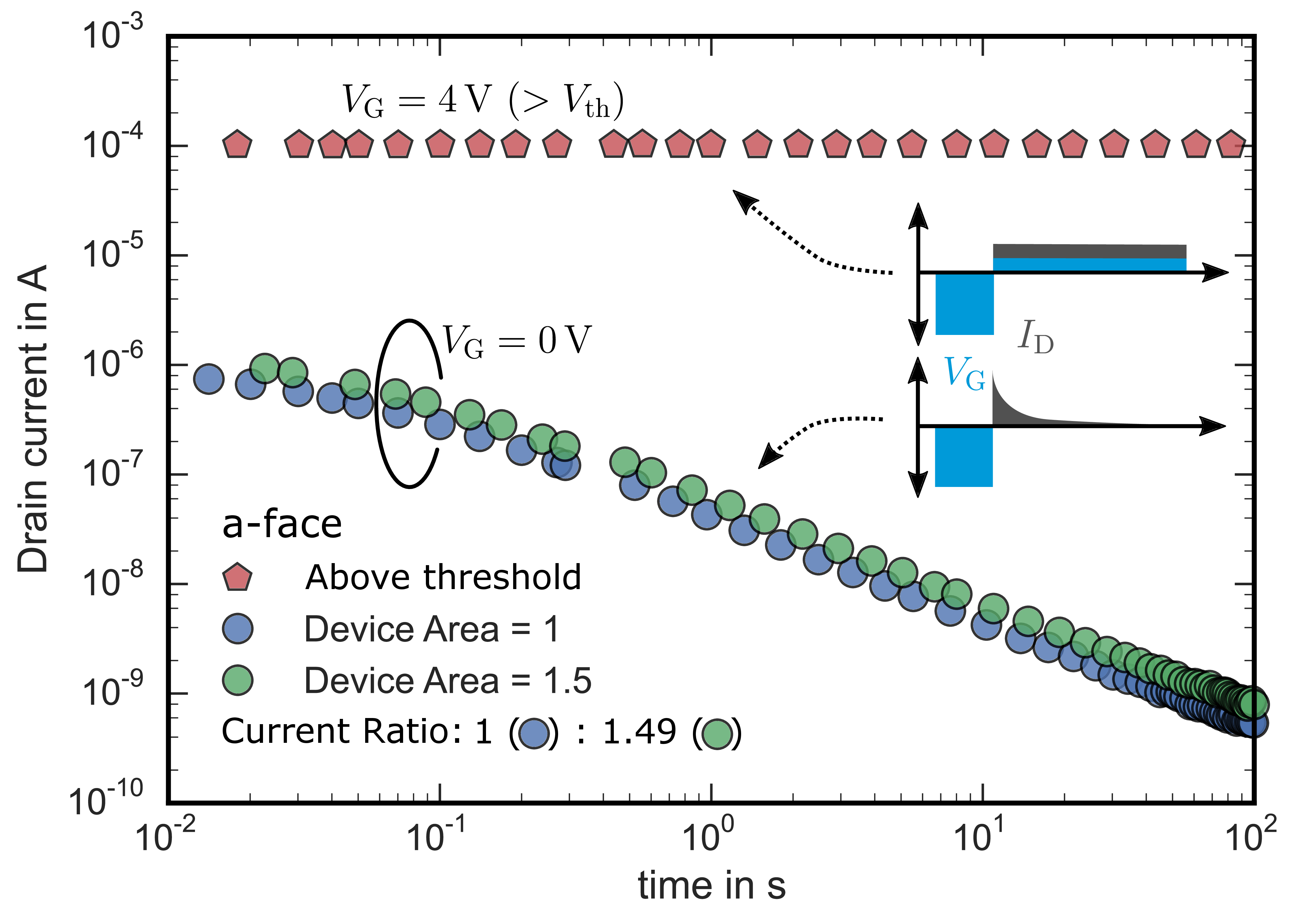
Figure 2.5: Recovery of the drain current at gate voltages below and above the threshold voltage after a charging pulse at $\ac {vg} = \SI {-15}{\V }$ for 1 s on an a-face device. The same trend is observed on Si-face devices. The green data corresponds to a device with a 50 % increased active area. The measurement pattern is depicted in the inset.

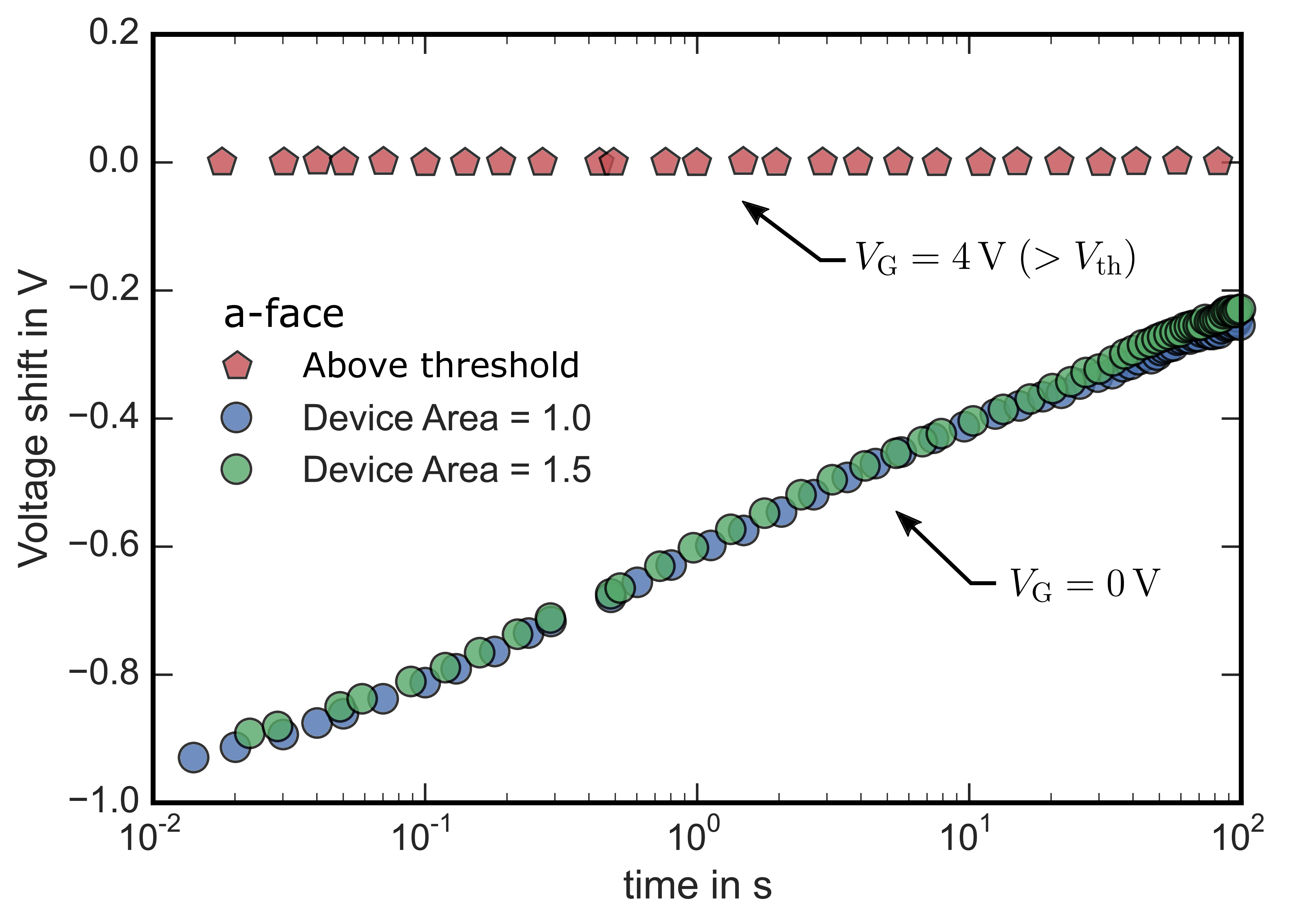
Figure 2.6: Voltage shift according to the recovery of the drain current in Fig. 2.5. The voltage shift is independent of the active area (circles) and disappears for gate voltages above the threshold voltage (red, pentagons) indicating a uniform distribution of the drain current over the entire active device area.

To analyze the time dependence of the hysteresis recovery, we start with the worst case scenario: a bias switch from strong accumulation with a completely positively charged interface to (a) the threshold voltage, or (b) 0 V. The outcome is shown in Fig. 2.5. For a bias switch from $\ac {vg} = \SI {-15}{\V }$ to $\ac {vg} = \SI {4}{\V }$ , which is slightly above the threshold voltage. In this case, (math image) is stable over time (red, pentagons) and the hysteresis has already fully recovered before the experimental time window, which starts 20 ms after the bias switch from $\ac {vg} = \SI {-15}{\V }$ to $\ac {vg} = \SI {4}{\V }$ . On the other hand, switching from $\ac {vg} = \SI {-15}{\V }$ to $\ac {vg} = \SI {0}{\V }$ results in measurable drain current transients due to the hysteresis effect (green and blue). Typically (math image) should be lower than 1 × 10⁻¹⁰ A at $\ac {vg} = \SI {0}{\V }$ . However, due to the negative shift of $\ac {Vsth}(\uparrow )$ caused by trapped positive charges, a drain current of approximately 1 µA is detected 20 ms after switching the bias from $\ac {vg} = \SI {-15}{\V }$ to $\ac {vg} = \SI {0}{\V }$ . The drain current transients are only visible due to the very slow detrapping of charges for Fermi level positions away from the SiC conduction band edge, which is the case for a gate voltage around 0 V.

From Fig. 2.5 it is also clear that the hysteresis does not change with the active device area. This is proven via the green and blue data traces (circles), which represent two a-face devices with different active area but otherwise identical. The drain current scales perfectly with device area indicating a uniform distribution of the current over the whole device. The corresponding voltage shift at $\ac {vg} = \SI {0}{\V }$ is shown in Fig. 2.6 and is identical for both devices. This proves that the hysteresis is not due to a local device leakage current caused by localized electric field variations, which might for example occur at the edges of the device, but is uniformly distributed over the entire active device area. The voltage shift at $\ac {vg} = \SI {0}{\V }$ recovers slowly with approximately 175 mV per decade in time. Detrapping until the drain current falls below the measurable window takes at least 1000 s.

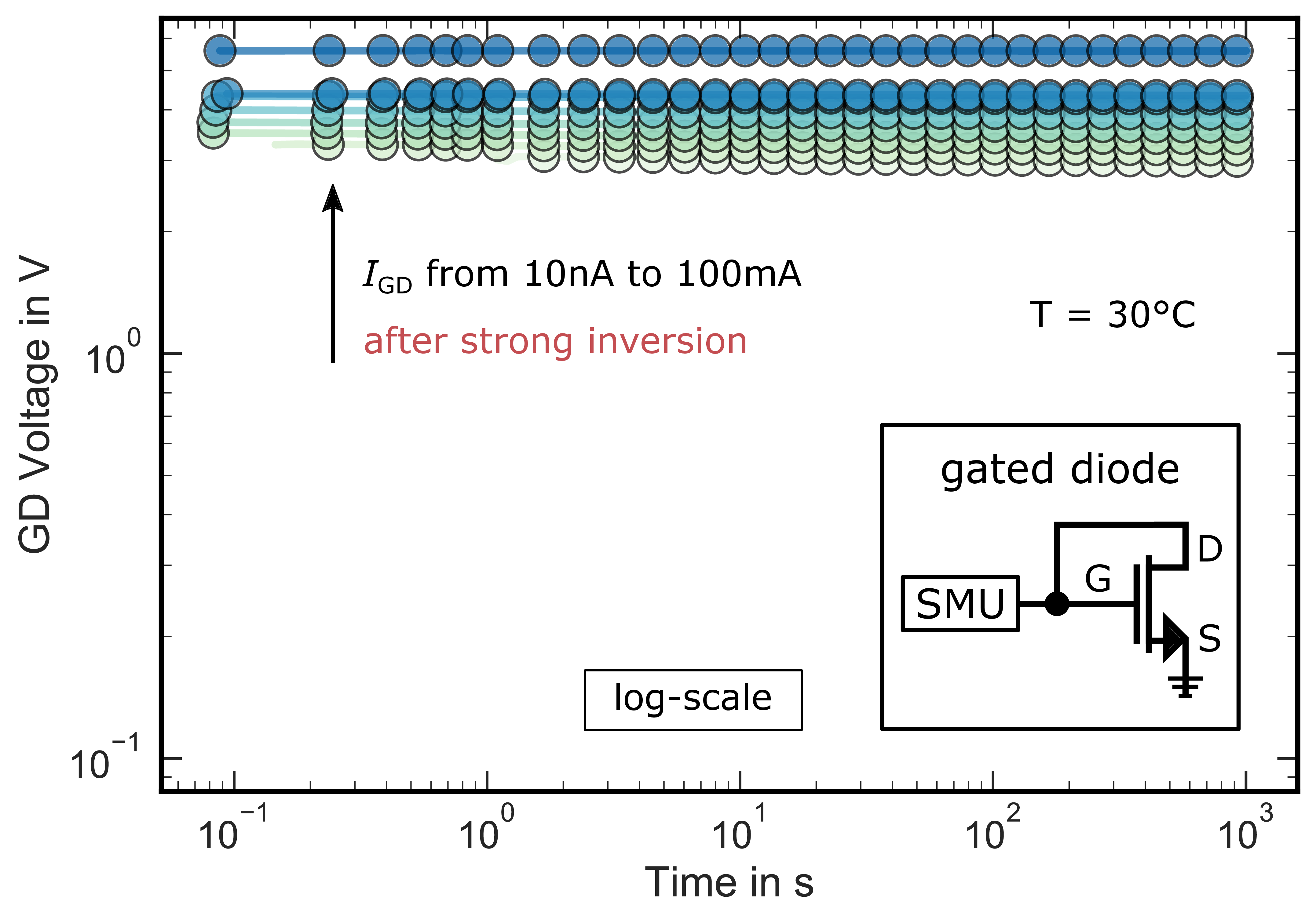
Figure 2.7: Measurement schematics for the extraction of the hysteresis using a gated diode circuit. The gate is either charged in strong-inversion (15 V) or in accumulation ( $-\SI {15}{\V }$ ) for a charging time of 1 s with grounded source and drain terminals (left). Afterwards, $\ac {VthGD}(\downarrow )$ or $\ac {VthGD}(\uparrow )$ , respectively, is extracted by forcing various current levels using the gated diode circuit with the drain con- nected to the gate contact.

$ -\SI {15}{\V } $ — Figure 2.7: Measurement schematics for the extraction of the hysteresis using a gated diode circuit. The gate is either charged in strong-inversion (15 V) or in accumulation ( $-\SI {15}{\V }$ ) for a charging time of 1 s with grounded source and drain terminals (left). Afterwards, $\ac {VthGD}(\downarrow )$ or $\ac {VthGD}(\uparrow )$ , respectively, is extracted by forcing various current levels using the gated diode circuit with the drain con- nected to the gate contact.

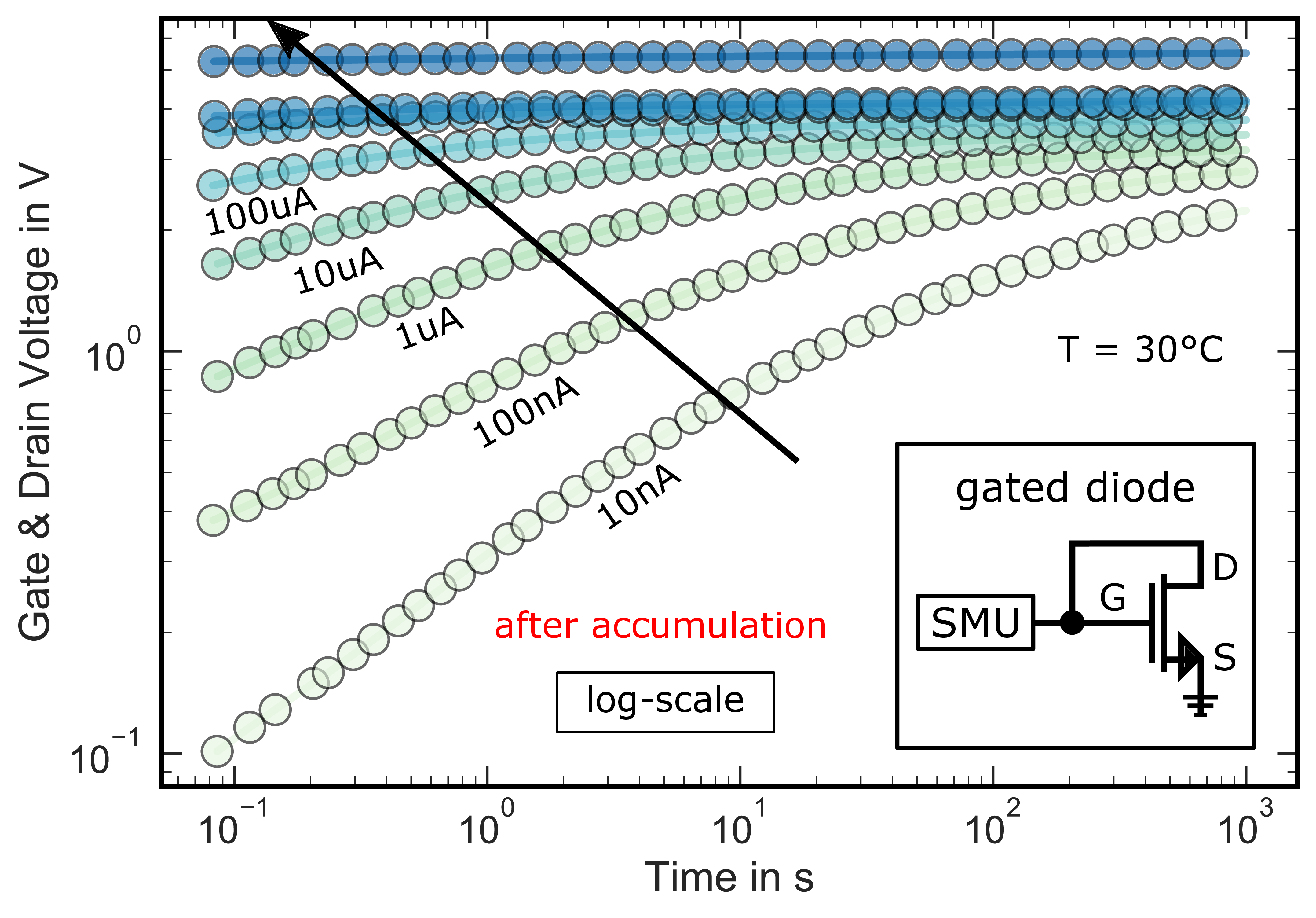
Figure 2.8: Gated-diode voltage $\ac {VthGD}(\downarrow )$ at various current levels ranging from 10 nA to 100 mA for 1000 s after charging the gate in strong-inversion at 15 V for 1 s. $\ac {VthGD}(\downarrow )$ is nearly stable within the measurement window. All traces have been recorded using a *gated diode* circuit, which shorts the gate and drain contacts of the devices as indicated in the inset.

$ \ac {VthGD}(\downarrow ) $ — Figure 2.8: Gated-diode voltage $\ac {VthGD}(\downarrow )$ at various current levels ranging from 10 nA to 100 mA for 1000 s after charging the gate in strong-inversion at 15 V for 1 s. $\ac {VthGD}(\downarrow )$ is nearly stable within the measurement window. All traces have been recorded using a *gated diode* circuit, which shorts the gate and drain contacts of the devices as indicated in the inset.

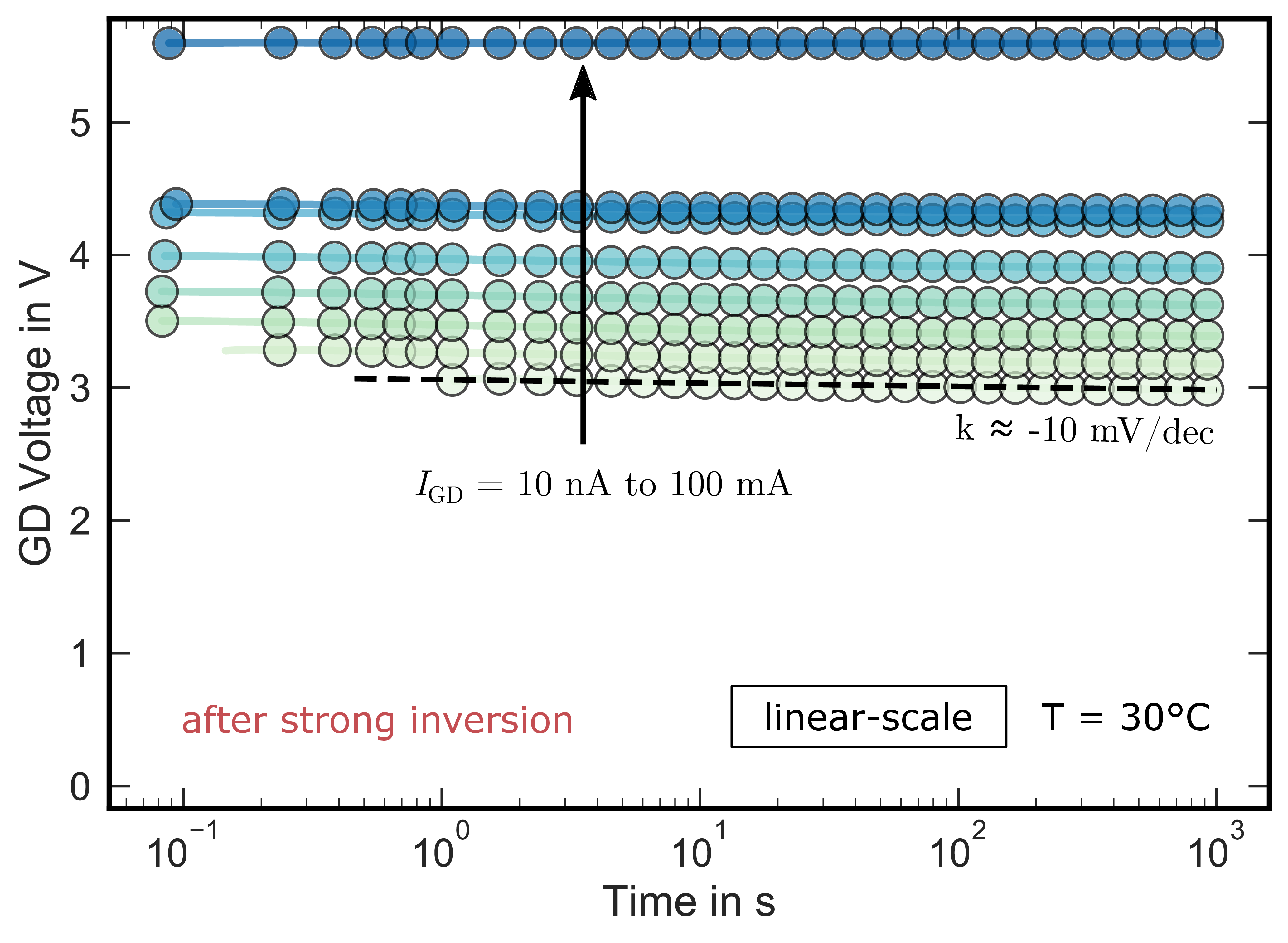
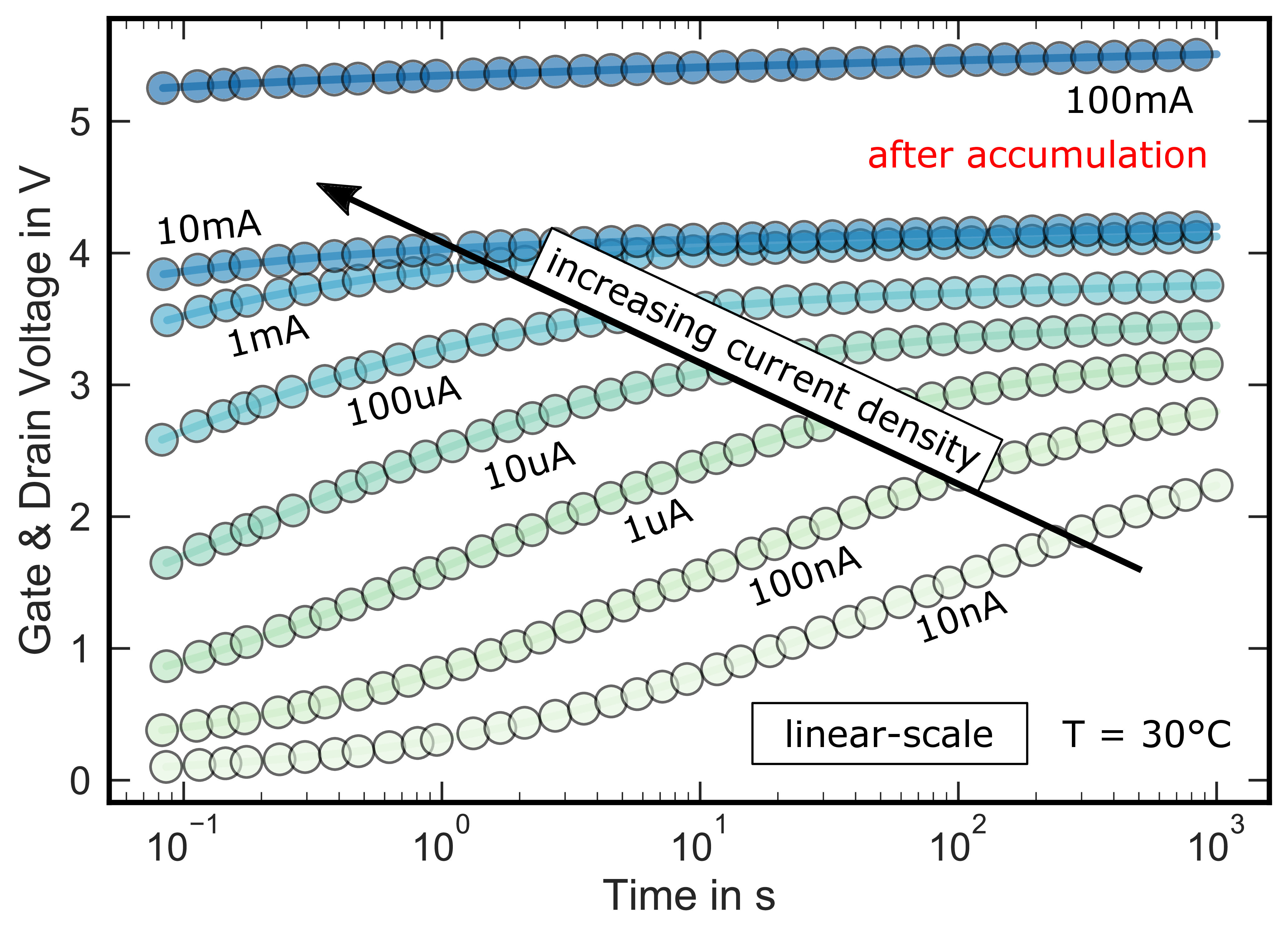
Figure 2.9: Same as Fig. 2.8 but now with linear y-scale. Only a small remaining recovery slope of approximately $\Delta \ac {VthGD}(\downarrow ) \approx -\SI {10}{\milli \V }/$ dec is visible due to charge emission form trap states, which captured an electron during the preceding inversion pulse.

$ \Delta \ac {VthGD}(\downarrow ) \approx -\SI {10}{\milli \V }/ $ — Figure 2.9: Same as Fig. 2.8 but now with linear y-scale. Only a small remaining recovery slope of approximately $\Delta \ac {VthGD}(\downarrow ) \approx -\SI {10}{\milli \V }/$ dec is visible due to charge emission form trap states, which captured an electron during the preceding inversion pulse.

2.4.1 Hysteresis after strong inversion

Before we discuss the more complicated hysteresis effect after an accumulation pulse in more detail, we start by analyzing the threshold voltage instability after a positive bias pulse above threshold (inversion). In this case, the gate bias is switched from strong inversion to a fixed readout current at which $\ac {Vth}^{\text {GD}}(\downarrow )$ is extracted. Here, the superscript GD indicates that a gated-diode circuit is used for the readout of the threshold voltage $\ac {Vth}^{\text {GD}}(\downarrow )$ to keep the inversion carrier density in the channel constant during the readout. The stress/measurement schematics are shown in Fig. 2.7. At first, the interface is charged in strong inversion at $\ac {vg}=\SI {+15}{\V }$ for 1 s while the source and drain terminals are grounded (left). Afterwards, the connection scheme is changed to the gated diode configuration using a switching matrix and a current (math image) is forced at the shorted drain/gate terminals while the corresponding voltage at these terminals, $\ac {VthGD}(\downarrow )$ , is monitored. Note that no gate-leakage current flows if a current is forced during the gated-diode configuration. Instead, the potential at the gate terminal rises until the inversion channel forms. From this point on, the forced (math image) flows exclusively from drain to source, as indicated by the red arrows in Fig. 2.7.

Fig. 2.8 and Fig. 2.9 shows the $\ac {VthGD}(\downarrow )$ transients in logarithmic and linear y-scale at various forced current levels (math image) ranging from 10 nA to 100 mA for 1000 s. $\ac {VthGD}(\downarrow )$ is immediately stable within our measurement window. Only a small remaining recovery slope of approximately $\Delta \ac {VthGD}(\downarrow ) \approx -\SI {10}{\milli \V }/$ dec is visible due to charge emission from border/oxide traps, which captured an electron during the preceding strong inversion pulse. This behavior is the typical voltage recovery, which occurs after positive bias stress as will be discussed in more detail in Chap. 3. A basic overview is provided in Section 3.

Figure 2.10: Schematic illustration of the interface charging state after switching the gate bias from strong inversion to inversion. The change in gate voltage is indicated at the top. The observed instability (bottom) is mainly defined by electron emission from trap states close to the conduction band edge (middle).

The interface trap charging state after the bias change from strong inversion to $\ac {VthGD}(\downarrow )$ is sketched in Fig. 2.10. The bias switch is indicated at the top, the interface charge state in the middle and the observed relative hysteresis at the bottom. After the bias switch, the Fermi level changes only slightly and remains close to the conduction band edge. Assuming only interface states, the time constant for electron emission (math image) according to Shockley-Read-Hall (SRH) [112, 113] is given by

$(2.5) \{begin}{align} \label {SH:eq:ten} \ac {ten} = \frac {1}{\ac {vthn}\ac {sigman}\ac {Nc}} \exp \left ( \frac {\ac {Ec}-\ac {Et}}{\ac {kb}\ac {T}} \right ) \{end}{align}$

with the thermal velocity for electrons (math image) , the capture cross section and the effective density of states in the conduction band . After the bias switch from strong inversion to inversion, remains close to and thermal equilibrium is restored almost immediately by electron emission from trap states above the Fermi level to (Fig. 2.10, middle) resulting in a stable, hysteresis free, drain current (Fig. 2.10, bottom). Note that no hole capture occurs because the interface remains inverted.

The remaining recovery slope of approximately $\Delta \ac {VthGD}(\downarrow ) \approx -\SI {10}{\milli \V }/$ dec originates from border trap states with broadly distributed emission time constants, which can be described according to (1.6) (see Section 1.2.1). These states will be intensively investigated in Chap. 3.

Figure 2.11: Schematic illustration of the interface charging state after switching the gate bias from accumulation to inversion. Top: timing of the gate voltage. Middle: after the 1 s accumulation pulse, the interface is fully positively charged. Bottom: after switching to inversion, more and more positive charge is lost via hole emission and electron capture leading to a decrease a recovery of the hysteresis.

2.4.2 Hysteresis after accumulation

In contrast to the behavior after charging the interface in strong inversion, the outcome changes drastically if the device is charged via an accumulation pulse. The switching process is sketched in Fig. 2.11 with the voltage level at the gate contact (top), the interface charging state (middle), and the relative hysteresis (bottom). Starting in accumulation, where the Fermi level is pinned to the valence band, the interface is assumed to be positively charged and in thermal equilibrium. After switching to inversion, where (math image) is measured, the Fermi level crossed nearly the entire SiC band gap, which results in a non-steady interfacial charge state. Thermal equilibrium is slowly restored by

• electron capture from the SiC conduction band, with time constants according to

$(2.6) \begin{equation} \label {SH:eq:tcn} \ac {tcn} = \frac {1}{\ac {vthn}\ac {sigman}\ac {ninv}} \end{equation}$

with the inversion charge density ,
• and hole emission to the SiC valence band edge , which follows

$(2.7) \{begin}{align} \label {SH:eq:tep} \ac {tep} = \frac {1}{\ac {vthp}\ac {sigmap}\ac {Nv}} \exp \left ( \frac {\ac {Et}-\ac {Ev}}{\ac {kb}\ac {T}} \right ) \{end}{align}$

with the thermal velocity and capture cross section of holes, and the effective density of states in the valence band [112, 113].

Figure 2.12: Voltage shift after switching the gate from accumulation to various current densities , ranging from 10 nA to 100 mA. transients are recorded using a gated-diode cir- cuit (inset).

(math image) — Figure 2.12: Voltage shift after switching the gate from accumulation to various current densities , ranging from 10 nA to 100 mA. transients are recorded using a gated-diode cir- cuit (inset).

Figure 2.13: Same as Fig. 2.12 but now with linear y-scale. Recovery of the subthreshold hysteresis scales with current density.

Since hole emission is independent of the Fermi level according to (2.7), the main contribution to the restoration of thermal equilibrium originates from electron capture according to (2.6). Therefore, the larger the inversion charge density (math image) (and thereby the gate voltage), the faster thermal equilibrium is reached. Fig. 2.12 and Fig. 2.13 show $\ac {VthGD}(\uparrow )$ for various inversion charge densities. As before, the measurement is performed according to the schematics sketched in Fig. 2.8. Instead of a strong-inversion pulse, an accumulation pulse at $\ac {vg}=\SI {-15}{\V }$ is applied for 1 s. After the charging pulse, (math image) ranging 10 nA to 10 mA is forced using the gated diode circuit, while the corresponding gate/drain voltage (labeled as $\ac {VthGD}(\uparrow )$ ) is monitored. From (2.6) and the fact that the drain current is to first order a direct measurement for via the textbook formula

$(2.8) \{begin}{align} \ac {id} = \frac {W}{L} \ac {mun} \ac {q}\ac {ninv} \ac {vd} , \{end}{align}$

it is obvious that the time to restore thermal equilibrium should be indirectly proportional to (math image) , if mainly interface states are responsible for the hysteresis. This is in fact the case, as will be shown within the next sections.

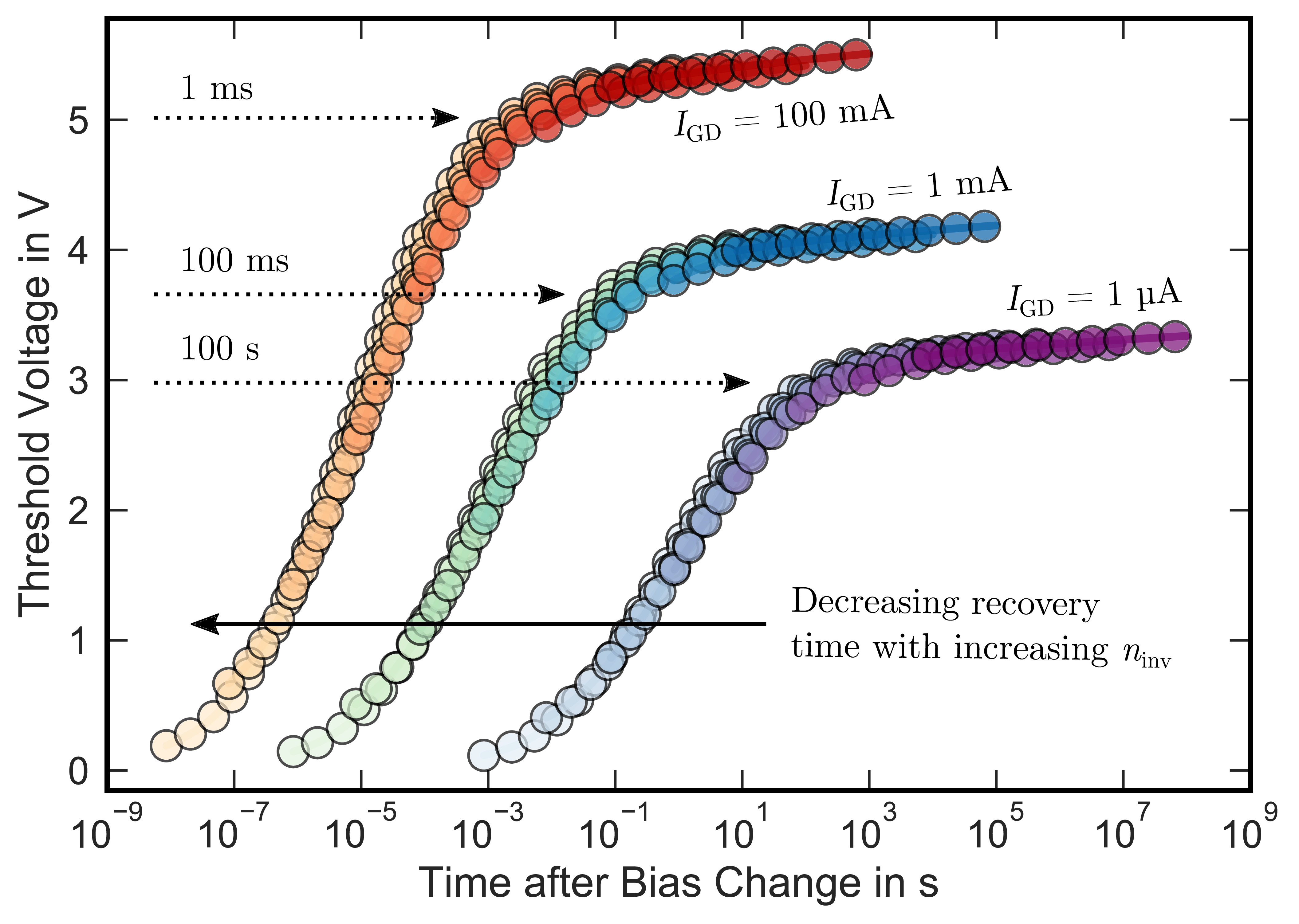
Figure 2.14: Universal recovery curve for $\ac {Ir}=\SI {1}{\milli \ampere }$ obtained by apply- ing (2.9) to the data in Fig. 2.12. At 1 mA the recovery of takes approximately 100 ms.

$ \ac {Ir}=\SI {1}{\milli \ampere } $ — Figure 2.14: Universal recovery curve for $\ac {Ir}=\SI {1}{\milli \ampere }$ obtained by apply- ing (2.9) to the data in Fig. 2.12. At 1 mA the recovery of takes approximately 100 ms.

Figure 2.15: Universal recovery curves for a readout current of 0.1 A (red), 1 mA (green), and 1 µA (purple). The recovery time scales linearly with the inversion carrier density . For $\ac {Igd} = \SI {1}{\milli \ampere }$ (blue), the re- covery time is approximately 100 ms, whereas for the 100 times higher current of $\ac {Igd} = \SI {100}{\milli \ampere }$ , the recovery time is 100 times shorter and approximately 1 ms (red).

Figure 2.16: Temperature dependence of the universal recovery curve at 1 mA for $\ac {T}=\SI {-40}{\celsius }$ (purple), $\ac {T}=\SI {30}{\celsius }$ (blue), and $\ac {T}=\SI {175}{\celsius }$ (red). The steady state $\ac {VthGD}\left (\ac {Igd}\right )$ decreases from around 4.6 V ( $\ac {T}=\SI {-40}{\celsius }$ ) to approximately 3.4 V ( $\ac {T}=\SI {175}{\celsius }$ ). At −40 °C there seems to be ongoing re- covery for recovery times >10 s.

$ \ac {T}=\SI {-40}{\celsius } $ — Figure 2.16: Temperature dependence of the universal recovery curve at 1 mA for $\ac {T}=\SI {-40}{\celsius }$ (purple), $\ac {T}=\SI {30}{\celsius }$ (blue), and $\ac {T}=\SI {175}{\celsius }$ (red). The steady state $\ac {VthGD}\left (\ac {Igd}\right )$ decreases from around 4.6 V ( $\ac {T}=\SI {-40}{\celsius }$ ) to approximately 3.4 V ( $\ac {T}=\SI {175}{\celsius }$ ). At −40 °C there seems to be ongoing re- covery for recovery times >10 s.

The universal hysteresis recovery curve

If the recovery time constants of the hysteresis mainly scales with the free inversion carrier density (math image) , an increase of by a fixed factor should decrease the recovery time constant by exactly the same factor according to (2.6). This is in fact true, since we are able to scale all transients from Fig. 2.12 onto a single universal recovery time $t_\text {D}^\text {u}$ at any given readout current (math image) , by assuming $t_\text {D}^\text {u}$ to scale according to

$(2.9) \{begin}{align} \label {SH:eq:uni_recovery} t_\text {D}^\text {u}(\ac {Ir}) = t_\text {D}(\ac {id}) \frac {\ac {Rch}(\ac {Ir})}{\ac {Rch}(\ac {id})} \approx t_\text {D}(\ac {id}) \frac {\ac {id}}{\ac {Ir}} \,\text {.} \{end}{align}$

Here, $t_\text {D}(\ac {id})$ is the time at the current level (math image) , and $\ac {Rch}(\ac {Ir})$ and $\ac {Rch}(\ac {id})$ the channel resistance at and , respectively.

Using the data from Fig. 2.12, all distinct transients can be transformed onto one universal recovery curve, which allows to extract the time to reach thermal equilibrium at any given inversion carrier density (math image) represented by . Fig. 2.14 shows the universal recovery curve for a drain current of $\ac {Ir} = \SI {1}{\milli \ampere }$ . Coming from strong accumulation, it takes about 100 ms until thermal equilibrium is restored and the corresponding gate voltage of 4 V to be stable. Fig. 2.15 shows the same curve for $\ac {Ir} = \SI {100}{\milli \ampere }$ , 1 mA and 1 µA. Since we are able to describe the time constants solely via the SRH model, the recovery time scales linearly with the drain current, meaning a 100 times higher drain current corresponds to 2 orders of magnitude faster recovery time constants. This is proven in Fig. 2.15: at 1 mA, the time to reach thermal equilibrium is approximately 100 ms, whereas for a 100 times higher drain current of 100 mA thermal equilibrium is reached in 1 ms, which is exactly 100 times faster. The same is true for a 1000 times lower current of 1 µA (purple).

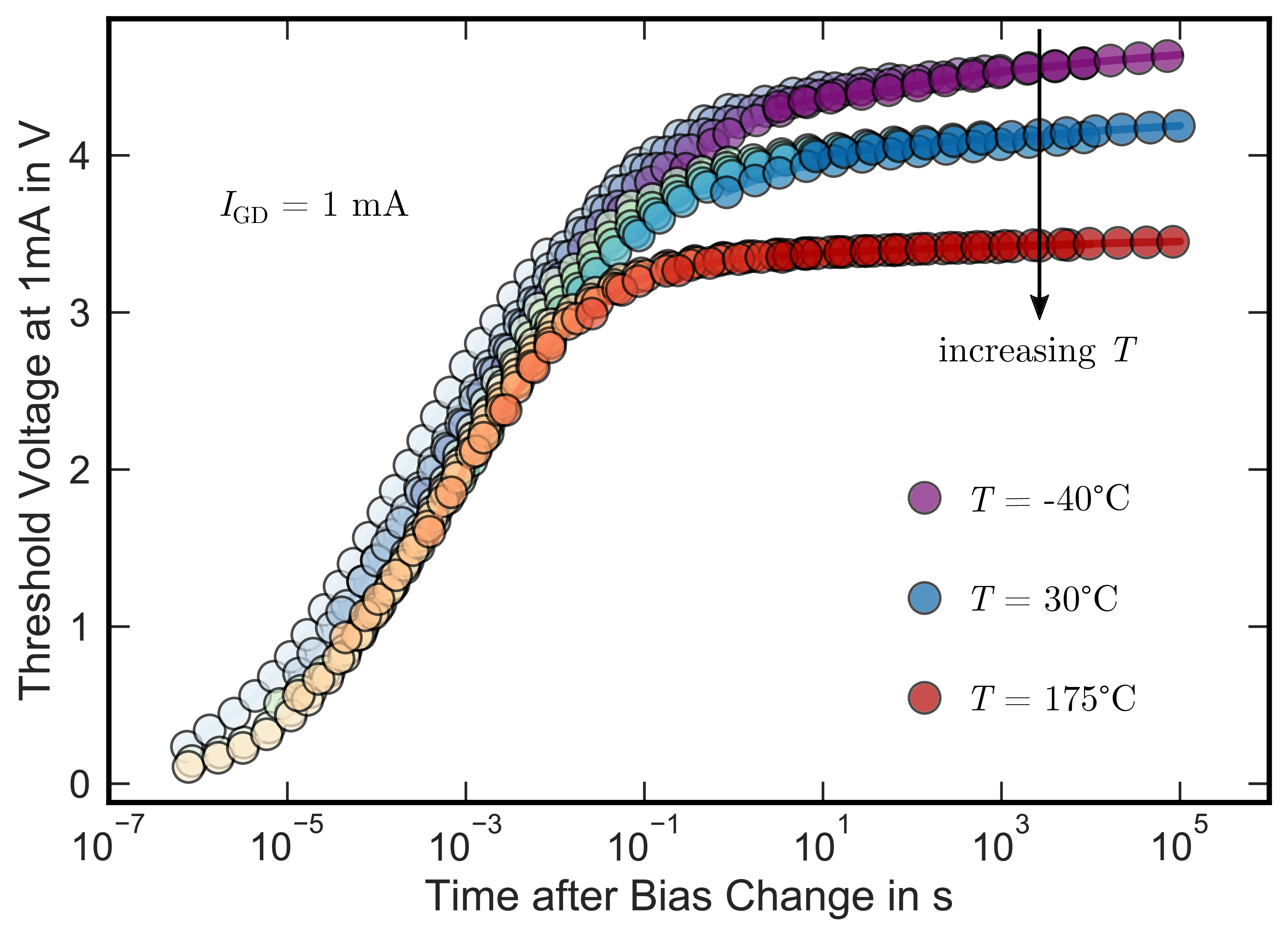
The temperature dependence of the universal recovery at $\ac {Igd} = \SI {1}{\milli \ampere }$ is shown in Fig. 2.16 for temperatures of −40 °C, 30 °C and 175 °C. As can be seen, the steady state $\ac {VthGD}\left ( \SI {1}{\milli \ampere } \right )$ decreases from 4.8 V to approximately 3.5 V with increasing temperature. Interestingly, there seems to be ongoing recovery at −40 °C and 30 °C for times $>\SI {10}{\s }$ , as can be seen in the remaining slopes of both curves. In general, one would attribute such a behavior to broadly distributed recovery time constants, which are hard to explain using a SRH based model. However, it will be shown in the next section that the ongoing recovery "tail" can be attributed to broadly distributed NBTI recovery and therefore decoupled from the hysteresis effect.

Distribution of recovery times

To extract the recovery time distribution of the interface states responsible for the hysteresis, first one has to disentangle the recovery signal from the convolution of fast states and the broadly distributed NBTI states. Fortunately, this is easily possible due to the broadly distributed emission times of border states after positive and negative bias stress, as was for example shown in Fig. 2.9 after positive bias temperature stress (PBTS) with a constant slope of approximately $k = \SI {-10}{\milli \V }/\text {dec}$ over the whole measurement window.

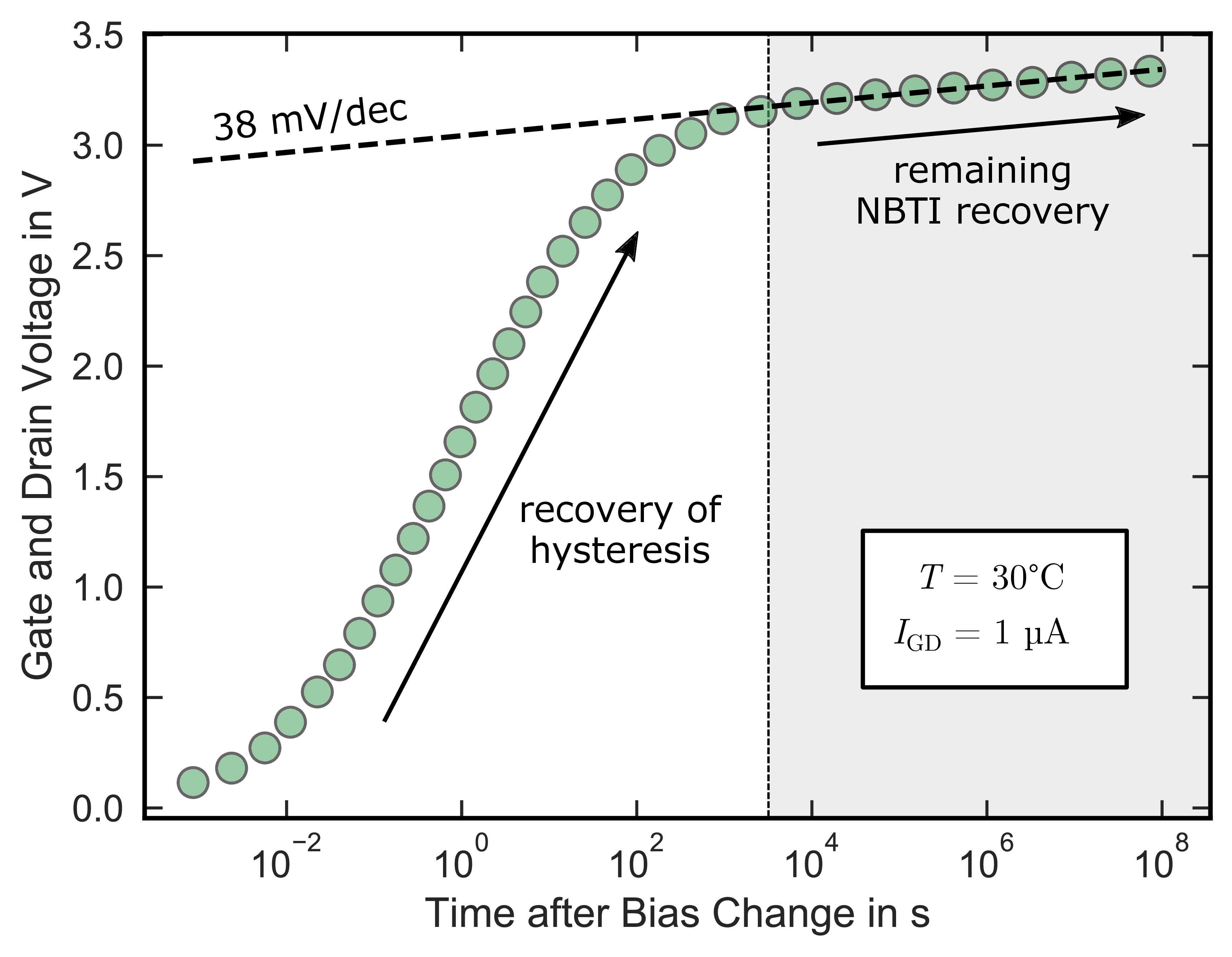
Figure 2.17: Convolution of the hysteresis recovery curve for a readout current of $\ac {Igd} = \SI {1}{\micro \ampere }$ . In addition to the recovery of fast states (white background), a minor contribution originates from border states with broadly distributed time constants (NBTI recovery). The NBTI recovery component becomes dominant after the hysteresis is fully recovered (gray background).

The convolution of the hysteresis recovery curve for a readout current of $\ac {Igd} = \SI {1}{\micro \ampere }$ is shown in Fig. 2.17. In addition to the dominant recovery component of the fast interface states responsible for the hysteresis, an additional NBTI recovery component is present, which becomes dominant at approximately 3 × 10³ s after the bias switch. Fortunately, the NBTI component is broadly distributed in time and therefore easy to remove from the hysteresis recovery curve using a constant exponent $k$ (cf. (1.3)), which allows for an explicit extraction of the recovery time distribution.

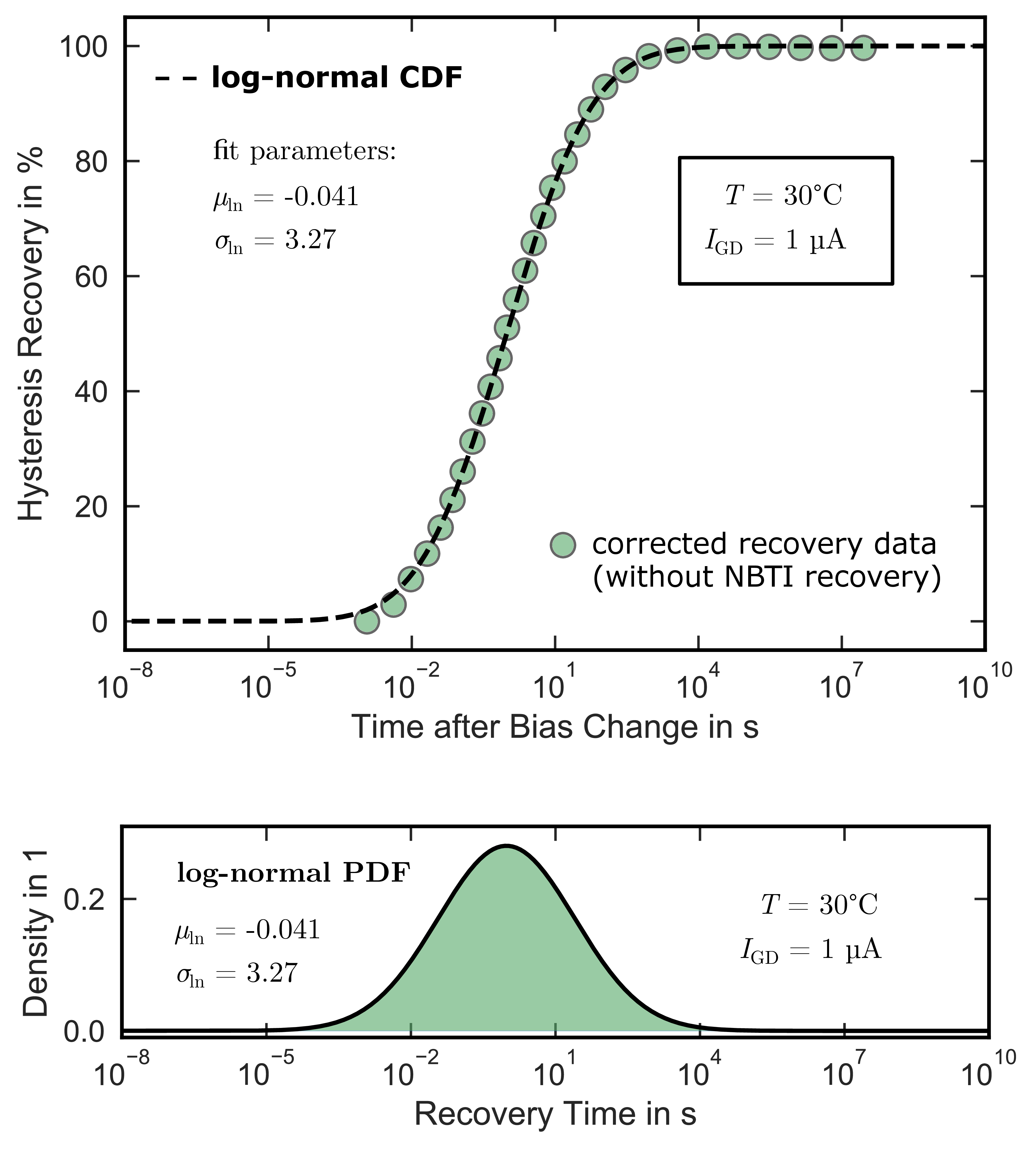
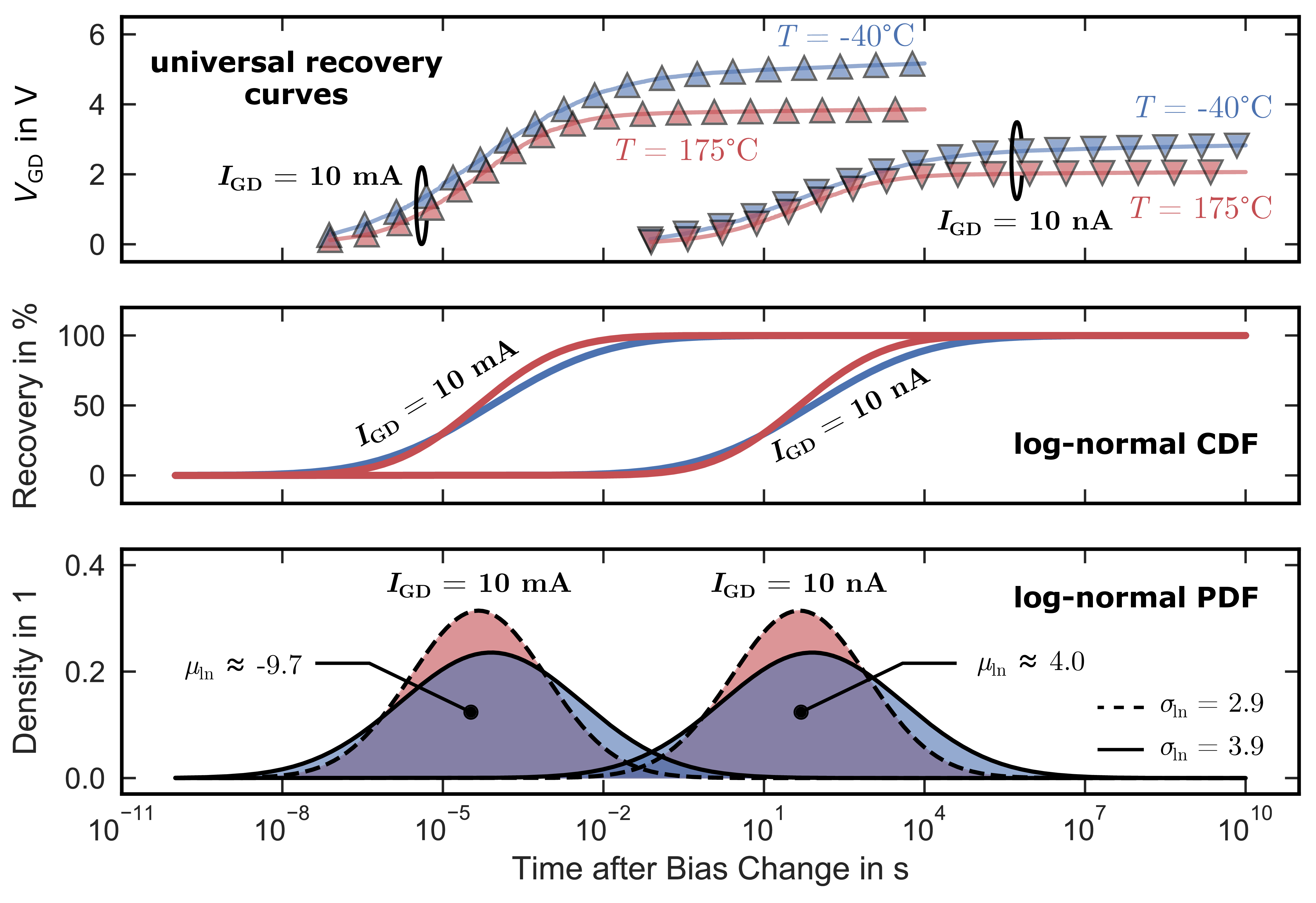
After the subtraction of the NBTI recovery, the recovery time (math image) of the hysteresis can be fitted using a log-normal distribution with the cumulative distribution function (CDF)

$(2.10) \{begin}{align} \text {\ac {CDF}}(\ac {trec}) = \frac {1}{2} + \frac {1}{2} \, \text {erf}\left ( \frac {\ln (\ac {trec}) - \ac {mu_ln} }{\sqrt {2} \ac {sigma_ln}} \right ) \{end}{align}$

with the error function erf, and the parameters of the log-normal distribution (math image) and that are, respectively, the mean and standard deviation of the normal distribution of $\log (\ac {trec})$ . The outcome for the universal recovery curve at $\ac {Igd} = \SI {1}{\micro \ampere }$ and 30 °C is shown in Fig. 2.18 (top). After the NBTI correction, the hysteresis recovery curve shows very good agreement with the log-normal CDF with $\ac {mu_ln} = -0.04$ and $\ac {sigma_ln} = 3.27$ (dashed line). The resulting probability density function (PDF) represents the distribution of the recovery time and is shown in the bottom plot in Fig. 2.18.

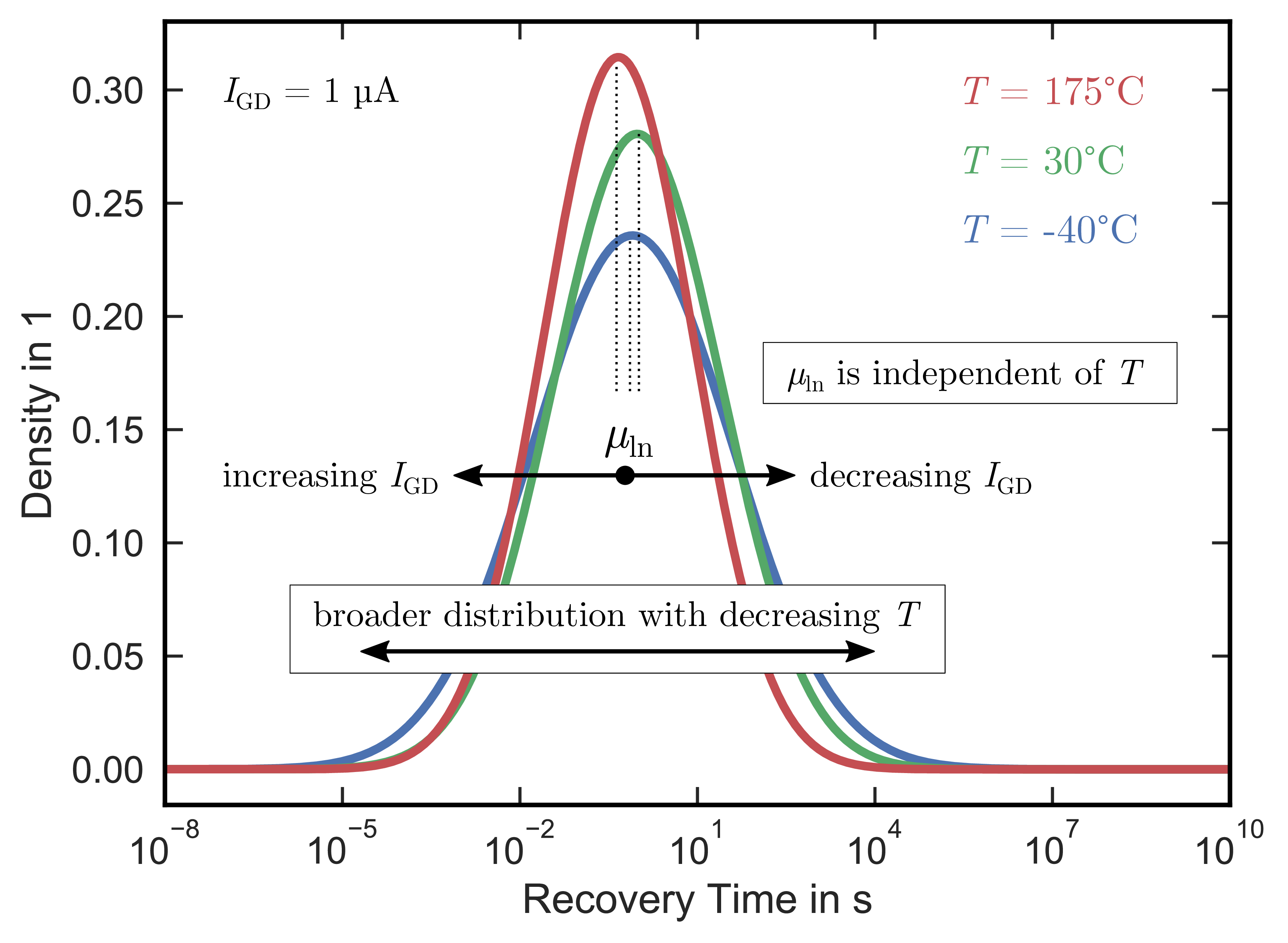
Figure 2.18: Recovery of the hysteresis at $\ac {Igd} = \SI {1}{\micro \ampere }$ and 30 °C after the removal of the NBTI related component (top, green circles). The recovery shows good agreement with a log-normal CDF with the fitting parameters $\ac {mu_ln} = -0.041$ and $\ac {sigma_ln} = 3.27$ . The representative PDF is indi- cated in the bottom plot.

Fig. 2.19 shows the log-normal PDFs for $\ac {T} = \SI {-40}{\celsius }$ (blue), $\ac {T} = \SI {30}{\celsius }$ (green) and $\ac {T} = \SI {175}{\celsius }$ (red) at a current density of $\ac {Igd} = \SI {1}{\micro \ampere }$ . The parameter (math image) is independent of the temperature and varies only slightly. Due to this, the recovery mechanism seems not to be temperature activated, or at least has an activation energy which is significantly below 100 meV. 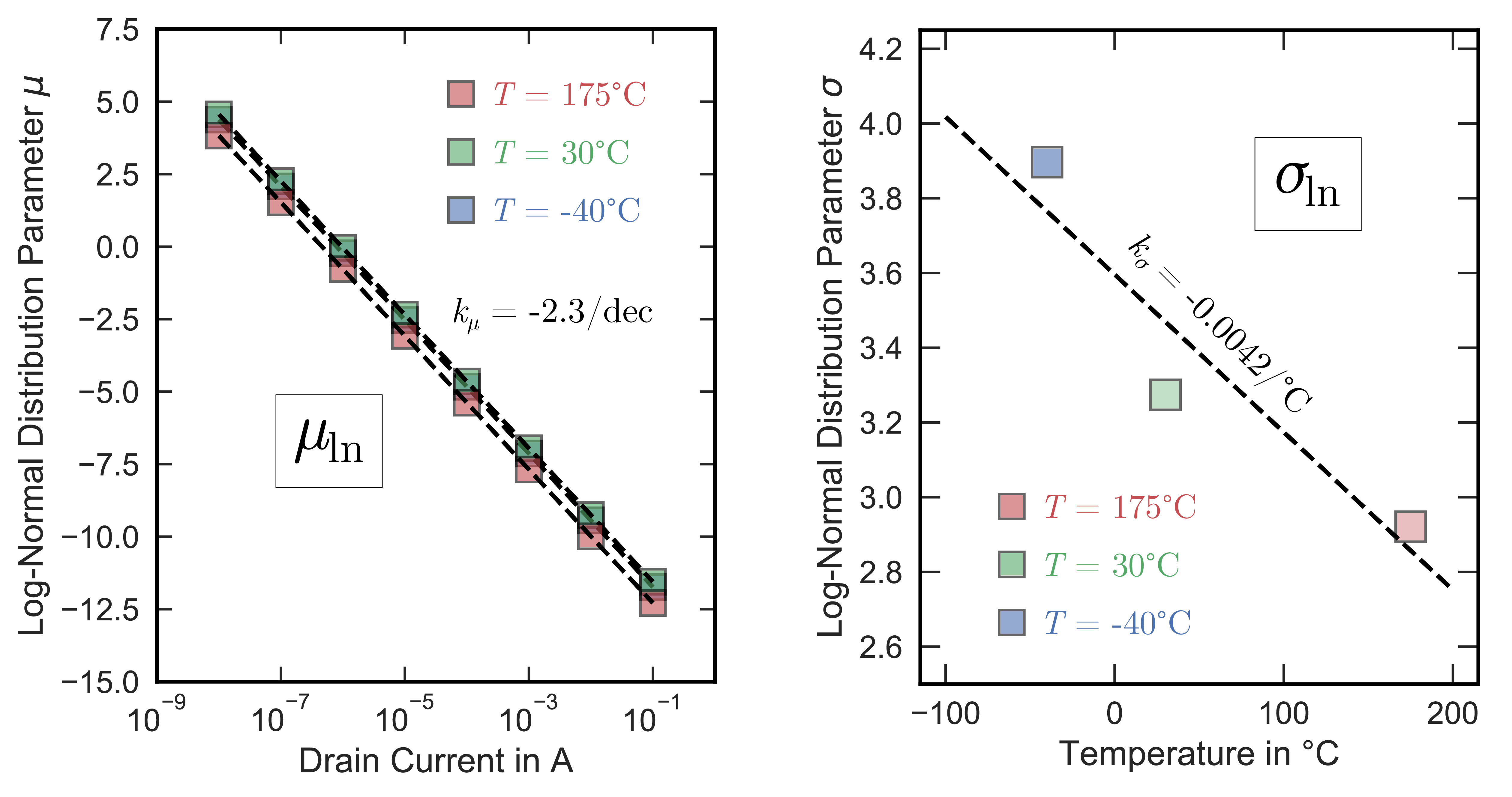On the other hand, the distribution broadens for decreasing temperatures, which results in slightly longer recovery times for lower temperatures. The dependence of the parameters of the log-normal distribution, (math image) and , on and is shown in Fig. 2.20. As already mentioned, is nearly independent of but scales strongly with the inversion carrier density (see Fig. 2.20, left), which is proportional to . The median of the distribution $\widetilde {\ac {trec}}$ , which is represented by the parameter (math image) according to

$(2.11) \{begin}{align} \widetilde {\ac {trec}} = \text {e}^{\ac {mu_ln}} \, \text {,} \{end}{align}$

decreases linearly with the logarithm of (math image) with a slope of $k_{\ac {mu_ln}} = -2.3 / \text {dec}$ . On the other hand, the standard deviation is not affected by (not shown), but increases with decreasing with a slope of $k_{\ac {sigma_ln}} \approx -0.004 / \SIUnitSymbolCelsius$ leading to a stretch out of the recovery time distribution for lower temperatures (Fig. 2.20, right).

Figure 2.19: Log-normal PDF for the hysteresis recovery times for $\ac {T} = \SI {-40}{\celsius }$ , $\ac {T} = \SI {30}{\celsius }$ and $\ac {T} = \SI {175}{\celsius }$ for $\ac {Igd} = \SI {1}{\micro \ampere }$ . If the temper- ature is decreased, the recovery time distribution broadens ( increases), whereas the parameter is roughly independent of within the measured temperature range.

Figure 2.20: Parameters for the log-normal distribution of the hysteresis recovery times. Left: the parameter seems to be independent of and scales linearly with $\log (\ac {Igd})$ with a slope of approximately $k_\mu = -2.3/ \text {dec}$ . Right: the standard devi- ation is independent of but seems to increase with decreasing , which indicates a broadening of the recovery time distribution for lower tempera- tures.

Figure 2.21: Recovery of the hysteresis as a function of the temperature for $\ac {T} = \SI {-40}{\celsius }$ (blue) and $\ac {T} = \SI {175}{\celsius }$ (red) for $\ac {Igd} = \SI {10}{\nano \ampere }$ (right) and a 1 million times higher current density of $\ac {Igd} = \SI {10}{\milli \ampere }$ (left). Top: universal recovery curves including the NBTI component, which is more pronounced for lower temperatures. Middle: log-normal cumulative distribution functions of the universal hysteresis curves corrected for NBTI recovery. Bottom: probability density functions of the recovery times. For the $10^6$ times higher current density (left), parameter decreases from $\ac {mu_ln} \approx 4$ to $\ac {mu_ln} \approx -9.7$ for both temperatures, which represents a $10^6$ times faster recovery time. On the other hand, the standard deviation increases from $\ac {sigma_ln} = 2.9$ at $\ac {T} = \SI {175}{\celsius }$ to $\ac {sigma_ln} = 3.9$ at $\ac {T} = \SI {-40}{\celsius }$ with decreasing tem- perature. This means the recovery time distribution broadens with lower and full recovery takes slightly longer.

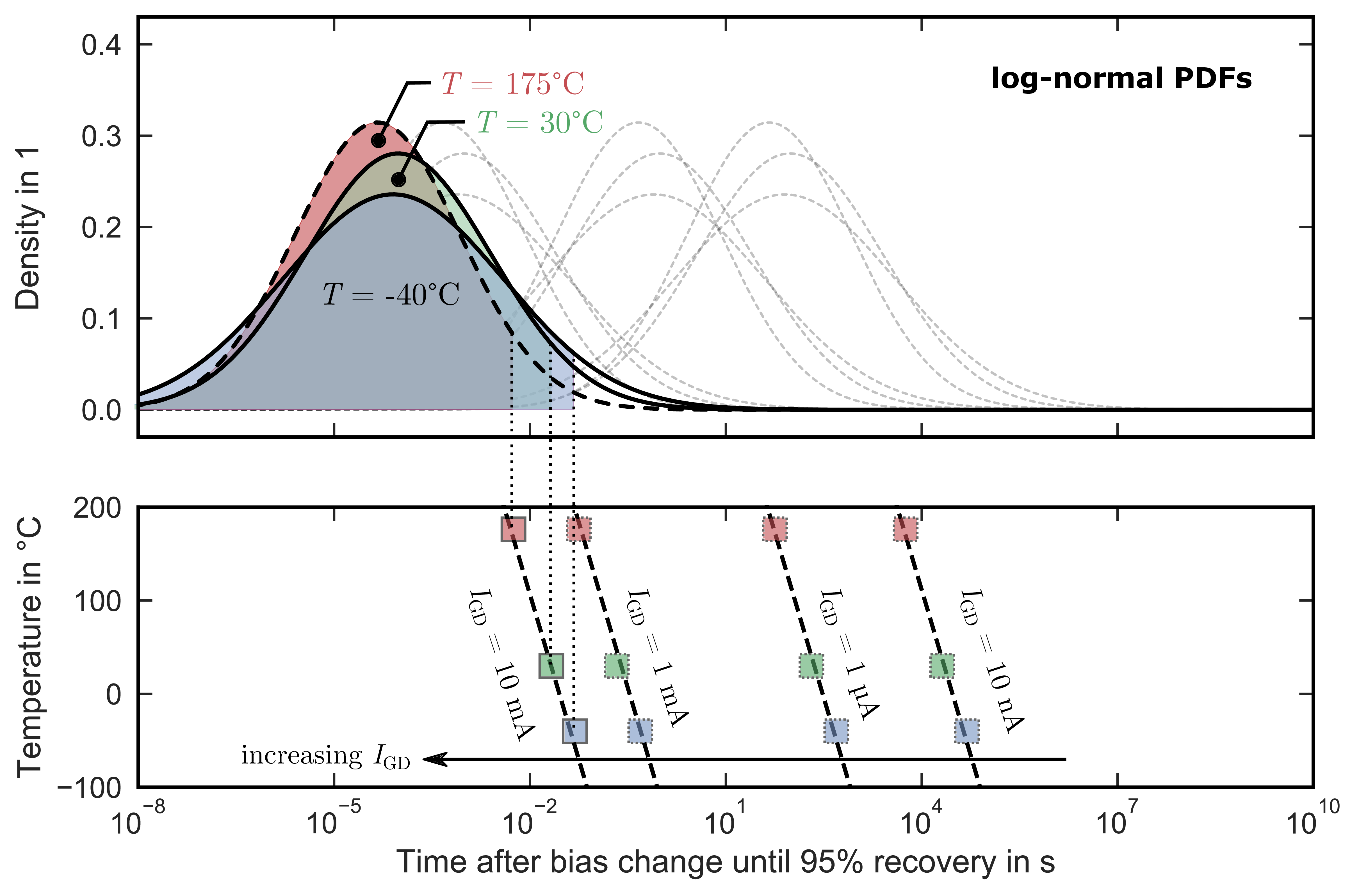
Figure 2.22: Time after bias change after which 95 % of the hysteresis is recovered for temperatures of $\ac {T} = \SI {-40}{\celsius }$ (blue), $\ac {T} = \SI {30}{\celsius }$ (green) and $\ac {T} = \SI {175}{\celsius }$ (red) and current densities ranging from $\ac {Igd} = \SI {10}{\nano \ampere }$ to $\ac {Igd} = \SI {10}{\milli \ampere }$ . Top: proba- bility density functions. Here the distributions for a current density of $\ac {Igd} = \SI {10}{\milli \ampere }$ are highlighted, whereas the PDFs for lower current densities are outlined only (dashed). Bottom: recovery time for 95 % recovery as a function of the temperature for various current densities.

Fig. 2.21 gives a final overview on the recovery of the hysteresis as a function of the temperature for $\ac {T} = \SI {-40}{\celsius }$ (blue) and $\ac {T} = \SI {175}{\celsius }$ (red) for two different current densities of $\ac {Igd} = \SI {10}{\nano \ampere }$ (right) and a 1 million times higher current density of $\ac {Igd} = \SI {10}{\milli \ampere }$ (left). Fig. 2.21, top, shows the universal recovery curves, still including the NBTI component. As stated before, this component is more distinct for lower temperates, as can be seen in the higher slope after the majority of the hysteresis is already recovered. After the NBTI component is removed, the relative amount of recovery can be extracted, which is represented by the CDF (Fig. 2.21, middle). From the CDF, one can extract the recovery time distributions (PDF, Fig. 2.21, bottom). The standard deviation is independent of (math image) , but decreases from $\ac {sigma_ln} = 3.9$ at $\ac {T} = \SI {-40}{\celsius }$ to $\ac {sigma_ln} = 2.9$ at $\ac {T} = \SI {175}{\celsius }$ with increasing temperature, which means a broadening of the PDF for lower temperatures. On the other hand, parameter is nearly independent of (math image) but scales strongly with . For the $10^6$ times higher current density (left), decreases from $\ac {mu_ln} \approx 4$ to $\ac {mu_ln} \approx -10$ for both temperatures, which results in an exactly $10^6$ times faster recovery.

Last but not least, Fig. 2.22 shows the recovery time after which 95 % of the fully-charged hysteresis is recovered for temperatures of $\ac {T} = \SI {-40}{\celsius }$ (blue) and $\ac {T} = \SI {175}{\celsius }$ (red) with current densities ranging from $\ac {Igd} = \SI {10}{\nano \ampere }$ to $\ac {Igd} = \SI {10}{\milli \ampere }$ . The top picture shows the representative recovery time distributions for all temperature and (math image) combinations. Here, the distributions for $\ac {Igd} = \SI {10}{\milli \ampere }$ are highlighted do increase readability, whereas the PDFs for lower current densities are outlined only (dashed, gray). The time for 95 % recovery of each - combination is represented in the bottom plot (squares). As already shown above, the recovery time decreases linearly with increasing current density. At fixed (math image) , the main contribution to the recovery time dependence for different temperatures originates from the increasing standard deviation for lower temperatures and not from a change of , which roughly remains stable within the investigated temperature range. Therefore, the underlying mechanism seems not to be temperature activated. For $\ac {Igd} = \SI {10}{\milli \ampere }$ , the time to 95 % recovery increases from $\ac {trec} \approx \SI {5}{\milli \s }$ at 175 °C to $\ac {trec} \approx \SI {50}{\milli \s }$ at −40 °C.

2.4.3 The hysteresis in the non-radiative multi-phonon picture

In general, the movement of the defect transition is modeled along a reaction path or coordinate using non-radiative multi-phonon transitions (NMP) (see Section 1.2.1). In an NMP transition, the defect state changes without excitation or emission of photons, meaning the required energies have to be supplied by phonons. A very comprehensive overview on non-radiative multiphonon theory can be found in [58].

Figure 2.23: Simplified schematic of the sweep hysteresis using the non-radiative multi-phonon view in the hole picture. The valence band (top) and conduction band (bottom) are indicated in blue and represent the neutral charge state of the system. The potential energy surface of the positive charge state ( $+$ ) is indicated in red. In strong inversion (left), the tran- sition from the positive charge state to the neutral charge state, $+ \rightarrow 0$ , is nearly barrier-free with $\ac {Ea}^{+ \rightarrow 0} \approx \SI {0}{\eV }$ . In depletion (middle), the energy of the neutral state is decreased by $\Delta E$ , which leads to broadly distributed time constants due to the non-zero and distributed energy barrier $\ac {Ea}^{0 \rightarrow +}$ . In accumulation (right), the energy barrier for the transition of the neutral charge state to the positive states is close to zero, $\ac {Ea}^{0 \rightarrow +} \approx \SI {0}{\eV }$ , which results in the nearly instantaneous charging times.

$ + $ — Figure 2.23: Simplified schematic of the sweep hysteresis using the non-radiative multi-phonon view in the hole picture. The valence band (top) and conduction band (bottom) are indicated in blue and represent the neutral charge state of the system. The potential energy surface of the positive charge state ( $+$ ) is indicated in red. In strong inversion (left), the tran- sition from the positive charge state to the neutral charge state, $+ \rightarrow 0$ , is nearly barrier-free with $\ac {Ea}^{+ \rightarrow 0} \approx \SI {0}{\eV }$ . In depletion (middle), the energy of the neutral state is decreased by $\Delta E$ , which leads to broadly distributed time constants due to the non-zero and distributed energy barrier $\ac {Ea}^{0 \rightarrow +}$ . In accumulation (right), the energy barrier for the transition of the neutral charge state to the positive states is close to zero, $\ac {Ea}^{0 \rightarrow +} \approx \SI {0}{\eV }$ , which results in the nearly instantaneous charging times.

An illustration of a simple defect model in the hole picture which describes the hysteresis is given in Fig. 2.23. Each energy state can be approximated by an adiabatic potential energy surface (PES), which usually is approximated by a parabolic form. In strong inversion (left), the energy of the positive charge state (+, red) is close to the valence band edge (blue). Due to the previous observations of very fast recovery times for (math image) close to , the transition from $+ \rightarrow 0$ has to be nearly barrier-free with $\ac {Ea}^{+ \rightarrow 0} \approx \SI {0}{\eV }$ . Therefore, all available trap states will be in the neutral configuration in strong inversion.

After changing the gate voltage from inversion to depletion (middle), the energy of the neutral state decreases by $\Delta E$ and transitions from the neutral to the positive charge state (+, red) become more and more likely.

In accumulation (right), the transition barrier for charging the positive charge state approaches zero, $\ac {Ea}^{0 \rightarrow +} \approx \SI {0}{\eV }$ , which results in nearly instantaneous charging times. Furthermore, all available trap states will be in the positive configuration in accumulation. Note that after completely charging the interface in accumulation, a gate bias switch back do depletion will result in the broadly distributed recovery time constants as observed in Section 2.4.2 due to non-zero and distributed energy barriers $\ac {Ea}^{0 \rightarrow +} > 0$ in depletion (middle).

« Previous Up Next »Contents
Previous: 2.3 Gate voltage dependence Top: 2 On the first Component: the Subthreshold Hysteresis Next: 2.5 Crystal face dependence