Atomistic Modeling

Atomistic modeling is used in an increasing number of projects, either to supplement information from TCAD modeling (band structure, material parameters) or in its own right, to better understand material properties and material interfaces (defects, etc.). Some important examples are listed below.

Material Modeling

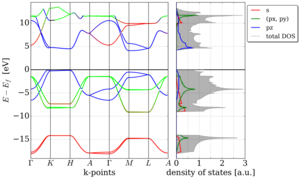
Fig. 1: Bandstructure of hBN as obtained from DFT

DFT is used to calculate the bandstructure of various materials for use in classical and quantum mechanical transport codes. This is particularly useful for the new class of 2D materials where material parameters are not easily accessible experimentally, see Fig 1. In addition, the properties of interfaces are explored for various material combinations.

Defect Modeling

Fig. 2: Volatility of a hydroxyl E' center caused by the release of hydrogen following the neutralization of the defect. On the right, the potential energy surface extracted from DFT is shown.

For reliability modeling, the properties of various point defects are thoroughly investigated using DFT. Recent investigations have shown that point defects in amorphous materials can have a multitude of possible states. One example is shown in Fig. 2, which demonstrates how hydrogen can be released from a hydroxyl E' center as soon as it becomes neutralized. Using DFT, the potential energy surfaces related to the various states are calculated, including nudged-elastic-band calculations to explore thermal barriers, which then form the basis for more efficient TCAD models.

Electromigration Modeling

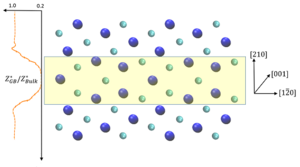
Fig. 3: Calculated values of the effective valence (Z*) for the case of reciprocal densities of the copper grain boundariesof coincident sites equal to 5 and tilt angle of 53.1 degrees (210)

The effective valence characterizes the susceptibility of the interconnect metal to electromigration and establishes a linear relationship between the electromigration force (with direct and electron wind components) and an external electric field. The applications of DFT, for metals carrying a steady state current, is of particular interest in calculation of the effective valence.

DFT calculations are used in combination with the non-equilibrium Green's function method to obtain non-equilibrium electron densities which allows for the determination of the atomic electromigration force (and thus, the effective valence) through application of the Feynman-Hellman theorem.